
Explain the Heisenberg Uncertainty Principle, and how the interpretation of it changes from the quantum to classical limits?
Answer
393.3k+ views
Hint: The Heisenberg uncertainty principle was given by the German physicist Werner Heisenberg in the year
Complete answer:
According to the Heisenberg Uncertainty Principle, if the uncertainty in the position of a wave-particle is small, the uncertainty in its momentum is large.
Mathematically, we could see that,
The Heisenberg Uncertainty Principle only applies to quantum objects like electrons and photons. Anything which is ordinarily-size has undetectable wave characteristics.
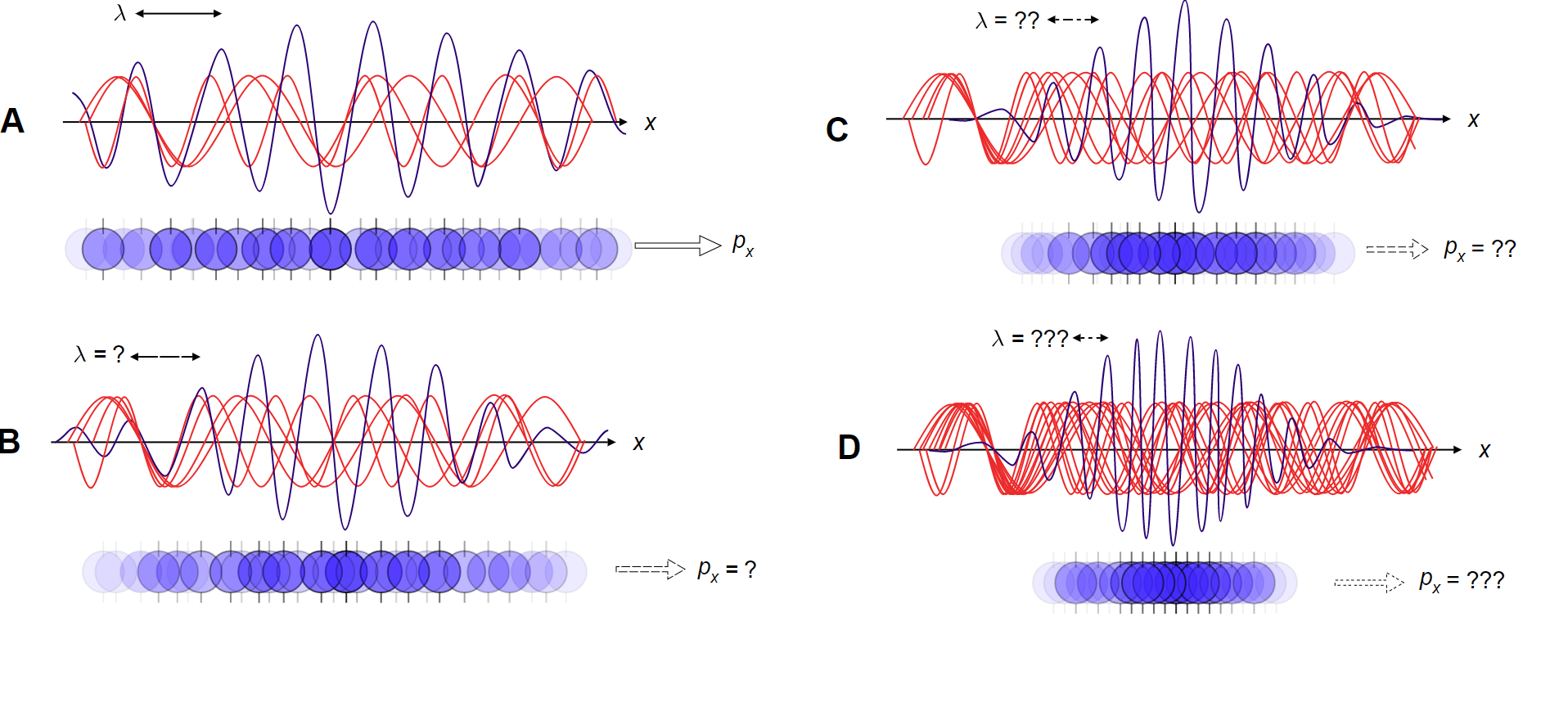
As we can see in the above image, in A; the wavelengths are well-defined. The momentum can be obtained from the wavelength, and so the momentum is also well-defined.
The correspondence principle states that in the limit as we stack more waves on the top of each other, ultimately the wavelengths become so small that the energy spectrum becomes continuous and we reach the classical limit, in which position is well-known.
Although, by doing this, we have layered so many waves that we have muddled them up amongst each other. Thus, we end up having a difficult time to refine the momentum via the wavelength.
Note:
If the position of the particles is the same, there would be an error in the momentum or the velocity or vice-versa. In the same way, the measurement of the momentum accurately will change the position. Thus, it proved that we cannot determine both position and momentum simultaneously.
Complete answer:
According to the Heisenberg Uncertainty Principle, if the uncertainty in the position of a wave-particle is small, the uncertainty in its momentum is large.
Mathematically, we could see that,
The Heisenberg Uncertainty Principle only applies to quantum objects like electrons and photons. Anything which is ordinarily-size has undetectable wave characteristics.

As we can see in the above image, in A; the wavelengths are well-defined. The momentum can be obtained from the wavelength, and so the momentum is also well-defined.
The correspondence principle states that in the limit as we stack more waves on the top of each other, ultimately the wavelengths become so small that the energy spectrum becomes continuous and we reach the classical limit, in which position is well-known.
Although, by doing this, we have layered so many waves that we have muddled them up amongst each other. Thus, we end up having a difficult time to refine the momentum via the wavelength.
Note:
If the position of the particles is the same, there would be an error in the momentum or the velocity or vice-versa. In the same way, the measurement of the momentum accurately will change the position. Thus, it proved that we cannot determine both position and momentum simultaneously.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




