
Find acceleration of centre of mass of the system as shown:
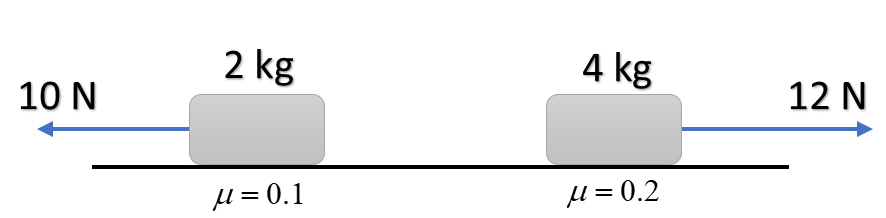

Answer
432.4k+ views
2 likes
Hint: Centre of the mass of the body is the point on which the whole mass is assumed to be concentrated. The concept of centre of mass is very important in solving mechanics problems. We can easily predict the trajectory of the motion of complex bodies. If the net force on the body is zero, then the center of mass of the body will not change its velocity, but it may be possible that some part of the body is undergoing complex rotational motion.
Formula used:
Complete answer:
The acceleration of centre of mass could be easily calculated using
The net external force on the block of 2kg
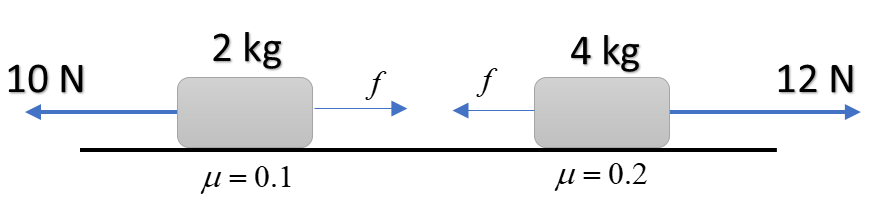
The net external force on the block of 10kg
Now, the net external force on the system is
Also, the total mass of the system is 2 kg + 4 kg = 6kg
Hence,
Thus
Hence the net acceleration of the centre of mass of the system is
Note:
Always note that the frictional force on a body is applied opposite to the direction of motion of the body. Here, one body is moving towards the left. Thus the direction of the force of friction is taken towards the right and the other is moving right, hence direction is taken towards left. In the final expression, acceleration is
Formula used:
Complete answer:
The acceleration of centre of mass could be easily calculated using
The net external force on the block of 2kg

The net external force on the block of 10kg
Now, the net external force on the system is
Also, the total mass of the system is 2 kg + 4 kg = 6kg
Hence,
Thus
Hence the net acceleration of the centre of mass of the system is
Note:
Always note that the frictional force on a body is applied opposite to the direction of motion of the body. Here, one body is moving towards the left. Thus the direction of the force of friction is taken towards the right and the other is moving right, hence direction is taken towards left. In the final expression, acceleration is
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




