
How do you find an equation of the straight line passing through the points with coordinates
Answer
402.3k+ views
Hint: Here, we are required to find the equation of a line passing through two given points. We will use the formula of the equation of a line which passes through the points
Complete step-by-step solution:
To solve his type of problem, we need to find the equation of line of pass through two points for that
We need to find the slope of line which is given by formula that is given by
For better understanding of the question figure is given below:
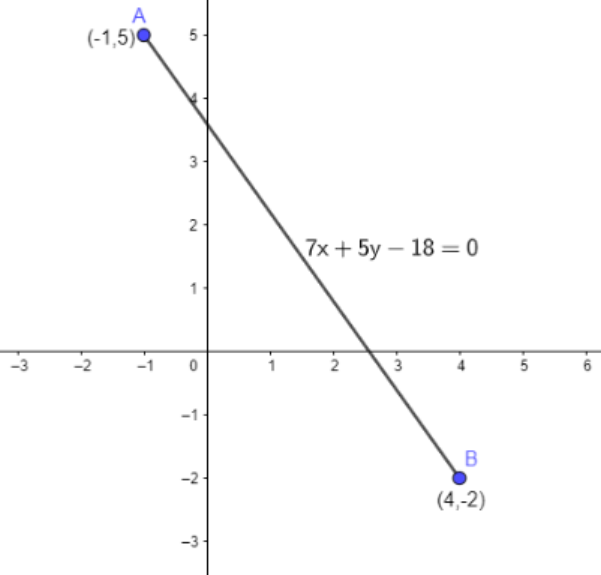
In this above figure, it has mentioned the line AB. We have to find the equation of line AB in such a way that the line passes through two points that are with coordinates
So, first of all we need to find the slope. When you notice this coordinates in the figure then you can see that
Formula is given by
Substitute the values in the above formula we get;
By simplifying this we get:
Now, we have to find the equation of a line using a given point and slope, we use the formula that is
Or we can write this as:
Substitute the value of equation (1) in equation (2) we get:
Hence the substituting the value of
By simplifying this we get:
By solving this bracket, we get:
Now, according to the question we have to write in the form of
To get in the form of equation, first of all we need to rearrange the term then we get:
In above equation multiply minus on both sides we get:
Hence, the equation of the line passing through the points
Note: In the standard form, an equation of a straight line is written as
When we are required to find an equation of a given line then, we use the relation between
Complete step-by-step solution:
To solve his type of problem, we need to find the equation of line of pass through two points for that
We need to find the slope of line which is given by formula that is given by
For better understanding of the question figure is given below:

In this above figure, it has mentioned the line AB. We have to find the equation of line AB in such a way that the line passes through two points that are with coordinates
So, first of all we need to find the slope. When you notice this coordinates in the figure then you can see that
Formula is given by
Substitute the values in the above formula we get;
By simplifying this we get:
Now, we have to find the equation of a line using a given point and slope, we use the formula that is
Or we can write this as:
Substitute the value of equation (1) in equation (2) we get:
Hence the substituting the value of
By simplifying this we get:
By solving this bracket, we get:
Now, according to the question we have to write in the form of
To get in the form of equation, first of all we need to rearrange the term then we get:
In above equation multiply minus on both sides we get:
Hence, the equation of the line passing through the points
Note: In the standard form, an equation of a straight line is written as
When we are required to find an equation of a given line then, we use the relation between
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




