
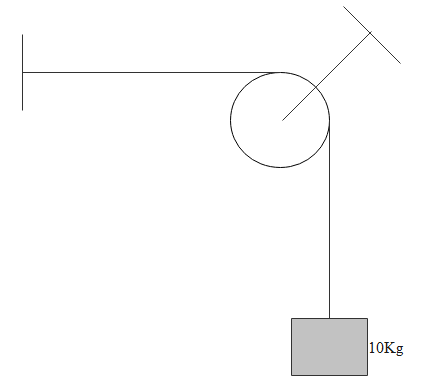
Find magnitude of force exerted by string on the pulley.
A.
B.
C.
D.

Answer
500.1k+ views
Hint: When a block is connected to the string and when it was given that the system is in equilibrium then here must be some force that balances the weight of the block and that force will be the tension and that will be equal to weight and tension is always a pulling force.
Formula used:
Complete answer:
The string used here is the same i.e the vertical portion of the string which connects the block and pulley and the horizontal portion of the string which connects the support and the pulley is the same. As long as the string is the same and massless the tension will be the same for both the portions. Since the system is in equilibrium the weight of the block is compensated by the tension in the string. So the weight of the block is a product of mass and acceleration due to gravity. Let the weight be W and mass be ‘m’ and acceleration due to gravity be ‘g’.

So the weight will be
Mass is given as 10Kg and acceleration due to gravity is
And the tension is ‘T’ is equal to the weight.
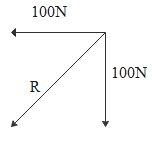
Since the angle between the two tensions is ninety degrees. The resultant will be
So, the correct answer is “Option B”.
Note:
The resultant of the tension forces will be the force acting on the pulley. That force will pass through the center of the pulley hence the torque produced by that force about the pulley center will be zero. Even though if we take the torques produced by the two forces individually the net torque will be zero.
Formula used:
Complete answer:
The string used here is the same i.e the vertical portion of the string which connects the block and pulley and the horizontal portion of the string which connects the support and the pulley is the same. As long as the string is the same and massless the tension will be the same for both the portions. Since the system is in equilibrium the weight of the block is compensated by the tension in the string. So the weight of the block is a product of mass and acceleration due to gravity. Let the weight be W and mass be ‘m’ and acceleration due to gravity be ‘g’.

So the weight will be
Mass is given as 10Kg and acceleration due to gravity is
And the tension is ‘T’ is equal to the weight.

Since the angle between the two tensions is ninety degrees. The resultant will be
So, the correct answer is “Option B”.
Note:
The resultant of the tension forces will be the force acting on the pulley. That force will pass through the center of the pulley hence the torque produced by that force about the pulley center will be zero. Even though if we take the torques produced by the two forces individually the net torque will be zero.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




