
Answer
462.6k+ views
Hint: First we will write the definition of the median in general and then we will write the given data and then we will make a more than curve and a less than curve by first making a more than cumulative frequency table and a less than cumulative frequency table, finally we will plot the obtained points and then where the two points intersect that will be our median.
Complete step by step answer:
First let’s understand the meaning of the median of a given data, so median is the middle value of the given set of data when arranged in a particular order. Now, if the number of observations in any given data is odd, therefore the median will be ${{\left( \dfrac{n+1}{2} \right)}^{th}}$term, whereas when the number of observations is even then the median will be both ${{\left( \dfrac{n}{2} \right)}^{th}}\text{ and }{{\left( \dfrac{n}{2}+1 \right)}^{th}}$ observations.
So, let’s consider the data given to us:
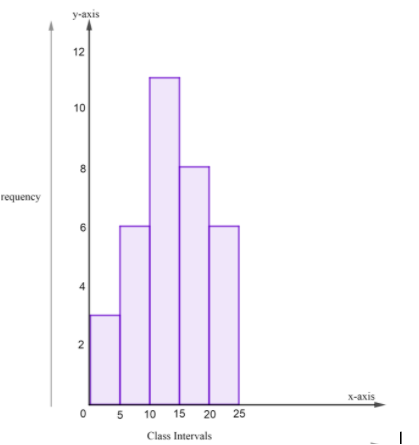
Graphical representation for above data will look like the following figure:
Similarly, we will form less than cumulative frequency table, that means we will take the upper limit of every class and see what is the total frequency present before that, which will be our cumulative frequency:
We will now plot these cumulative frequencies on a graph and form a more than curve and a less than curve and we know that the point where more than and less than curves meet then that will be our median.
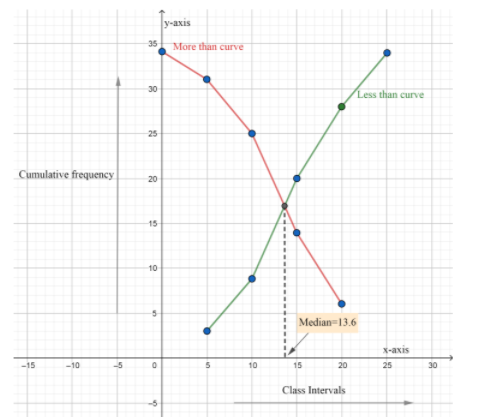
As, we see from the graph that more than and less than curve meets at 13.6 therefore, the median is 13.6
Note: Since, it is a grouped data conventional formula for median is not applied. For calculating the median of a grouped data, following formula is applied:
$\text{Median}=L+\dfrac{\left( \dfrac{n}{2} \right)-B}{G}\times w$
Where, L is the lower class boundary of the group containing the median, n is the total number of values, B is the cumulative frequency of the groups before the median group, G is the frequency of the median group and w is the group width.
Complete step by step answer:
First let’s understand the meaning of the median of a given data, so median is the middle value of the given set of data when arranged in a particular order. Now, if the number of observations in any given data is odd, therefore the median will be ${{\left( \dfrac{n+1}{2} \right)}^{th}}$term, whereas when the number of observations is even then the median will be both ${{\left( \dfrac{n}{2} \right)}^{th}}\text{ and }{{\left( \dfrac{n}{2}+1 \right)}^{th}}$ observations.
So, let’s consider the data given to us:
| Class Interval | Frequency |
| 0-5 | 03 |
| 5-10 | 06 |
| 10-15 | 11 |
| 15-20 | 08 |
| 20-25 | 06 |
Graphical representation for above data will look like the following figure:

Similarly, we will form less than cumulative frequency table, that means we will take the upper limit of every class and see what is the total frequency present before that, which will be our cumulative frequency:
| Class Interval | Cumulative Frequency |
| Less than 5 | 3 |
| Less than 10 | 9 |
| Less than 15 | 20 |
| Less than 20 | 28 |
| Less than 25 | 34 |
We will now plot these cumulative frequencies on a graph and form a more than curve and a less than curve and we know that the point where more than and less than curves meet then that will be our median.

As, we see from the graph that more than and less than curve meets at 13.6 therefore, the median is 13.6
Note: Since, it is a grouped data conventional formula for median is not applied. For calculating the median of a grouped data, following formula is applied:
$\text{Median}=L+\dfrac{\left( \dfrac{n}{2} \right)-B}{G}\times w$
Where, L is the lower class boundary of the group containing the median, n is the total number of values, B is the cumulative frequency of the groups before the median group, G is the frequency of the median group and w is the group width.
Recently Updated Pages
How is abiogenesis theory disproved experimentally class 12 biology CBSE

What is Biological Magnification

Fill in the blanks with suitable prepositions Break class 10 english CBSE

Fill in the blanks with suitable articles Tribune is class 10 english CBSE

Rearrange the following words and phrases to form a class 10 english CBSE

Select the opposite of the given word Permit aGive class 10 english CBSE

Trending doubts
Which are the Top 10 Largest Countries of the World?

What is the definite integral of zero a constant b class 12 maths CBSE

What are the major means of transport Explain each class 12 social science CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Explain sex determination in humans with the help of class 12 biology CBSE

How much time does it take to bleed after eating p class 12 biology CBSE




