
How do you find missing sides and angles of a non-right triangle, triangle ABC, angle C is 115, side b is 5, side c is 10?
Answer
477.6k+ views
Hint: In this question, we have to find the rest of the missing sides and angles. We will use the sine rule to find all the missing terms as we are given two sides and one angle in this question.
Law of sine:
Complete step by step answer:
Let’s solve the question.
First, understand how the law of sine works on triangles.
For any triangle:
In this figure, a, b, c are sides, and A, B and C are the angles.
So, it says that when we divide side ‘a’ by the sine of
Now, make a figure to see what all terms are given.
According to the law of sine:
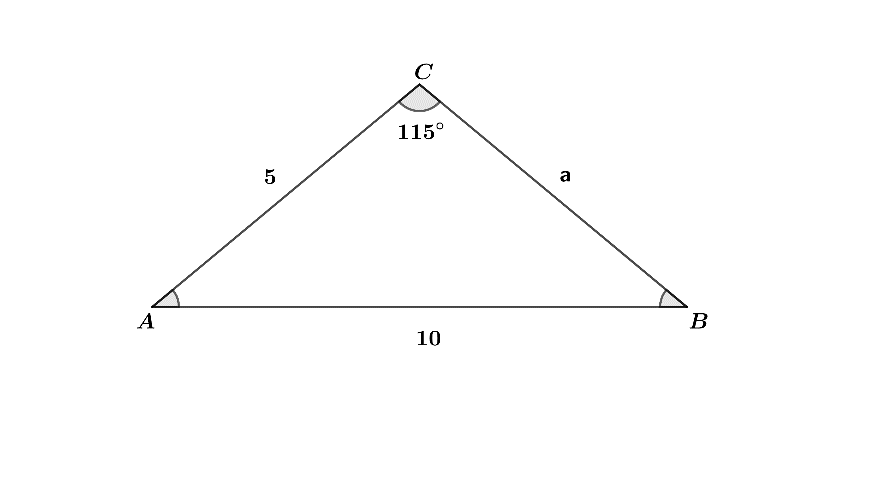
As, b = 5, c = 10 and
So we have to equate
Now put the values:
Now, leave sin B alone.
Now, use the inverse function of sine.
So, B = 26.95
Now, by angle sum property of a triangle, the sum of three angles of a triangle is
Put the value of B = 26.95 and
Now, we have to find side ‘a’.
As we know:
Now, let’s equate
Now, put the value c = 10,
After simplifying we get:
So, our final answer is: a = 6.8,
Note:
Students should know the law of sine for this question. A mistake can be made while applying inverse sine function here
Law of sine:
Complete step by step answer:
Let’s solve the question.
First, understand how the law of sine works on triangles.
For any triangle:
In this figure, a, b, c are sides, and A, B and C are the angles.

So, it says that when we divide side ‘a’ by the sine of
Now, make a figure to see what all terms are given.
According to the law of sine:

As, b = 5, c = 10 and
So we have to equate
Now put the values:
Now, leave sin B alone.
Now, use the inverse function of sine.
So, B = 26.95
Now, by angle sum property of a triangle, the sum of three angles of a triangle is
Put the value of B = 26.95 and
Now, we have to find side ‘a’.
As we know:
Now, let’s equate
Now, put the value c = 10,
After simplifying we get:
So, our final answer is: a = 6.8,
Note:
Students should know the law of sine for this question. A mistake can be made while applying inverse sine function here
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




