
Find out the quadrant of the point which divides internally the line segment joining (3, 4) and (7,-6) in two parts where one part is twice longer than the other.
(a)
(b)
(c)
(d)
Answer
504.9k+ views
Hint: A point can divide the line segment either internally or externally. If the point is on the line segment then the point divides the line segment internally at any particular ratio. The line is going to cut the line segment only internally, we only need to take care of the internal case using the section formula.
Complete step-by-step solution:
We know from section formula that if any point
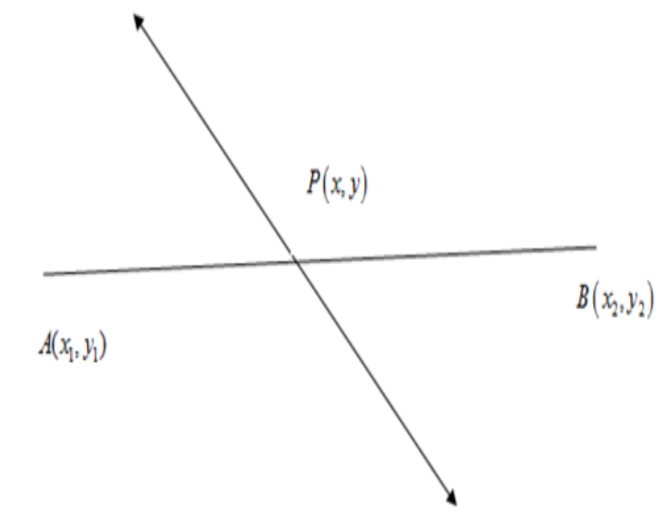
Let us assume that the line divides the line segment internally at the point
Case 1:
If the line divides in ratio
As
Case 2:
If the line divides in ratio 1:2 or PB is twice AP , then
As both
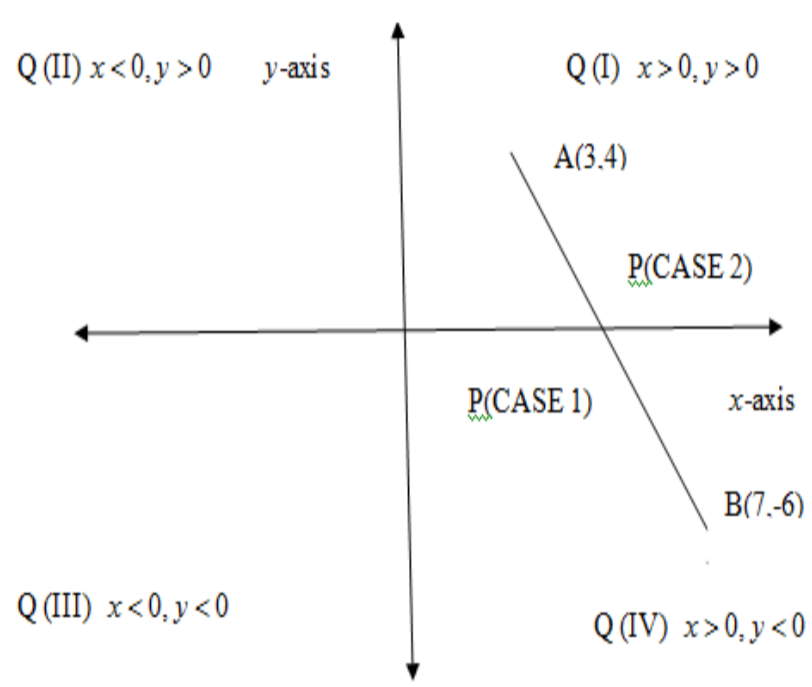
We can observe from the above image the two possible positions in two different quadrants of P in the
Note: We need to be careful to choose sectional formula from the confusion of internal and external division of a line segment. As the question is ambiguous about the ratio of division, it makes two cases. It more than one option correct type of MCQ.
Complete step-by-step solution:
We know from section formula that if any point

Let us assume that the line divides the line segment internally at the point
Case 1:
If the line divides in ratio
As
Case 2:
If the line divides in ratio 1:2 or PB is twice AP , then
As both

We can observe from the above image the two possible positions in two different quadrants of P in the
Note: We need to be careful to choose sectional formula from the confusion of internal and external division of a line segment. As the question is ambiguous about the ratio of division, it makes two cases. It more than one option correct type of MCQ.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




