
Find the acceleration of the block of mass M in the situation of figure. The coefficient of friction between the two blocks is
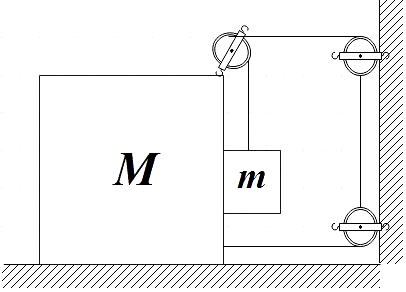

Answer
482.1k+ views
Hint: For the problems involving mass-pulley system, first we have to find all the accelerations acting on each of the given bodies. Then we have to draw the free body diagrams for each mass separately. Now by balancing the forces in both vertical and horizontal directions and then doing the necessary substitutions accordingly gives you the required acceleration.
Complete answer:
Let us assume that the body of mass M is accelerated towards the right with acceleration
Now that we have determined all the accelerations on the given masses, let us make free-body diagrams for each of the masses. The free-body diagram of mass m is given by,
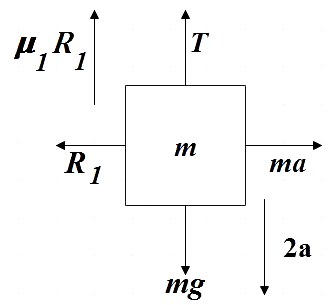
We see that the forces acting on mass m are: frictional force between two surfaces, weight downward, tension upward, normal reaction towards left, force due acceleration towards right and force due acceleration 2a downward. Now by balancing the forces in the vertical direction, we get,
But,
Free body diagram of mass M:
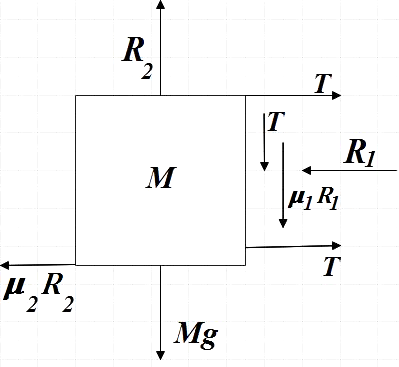
Balancing the vertical forces on M, we get,
Balancing the horizontal forces on M gives,
Substituting (2),
Substituting (1),
Hence we get the acceleration of the block of mass M as,
Note:
While drawing the free body diagrams, make sure that you don’t miss any of the forces acting on the masses. While marking the accelerations on the bodies keep in mind that you are dealing with a string constraint system. Also, chances are that you may miss normal forces while substituting, so take care of that too.
Complete answer:
Let us assume that the body of mass M is accelerated towards the right with acceleration
Now that we have determined all the accelerations on the given masses, let us make free-body diagrams for each of the masses. The free-body diagram of mass m is given by,

We see that the forces acting on mass m are: frictional force between two surfaces, weight downward, tension upward, normal reaction towards left, force due acceleration towards right and force due acceleration 2a downward. Now by balancing the forces in the vertical direction, we get,
But,
Free body diagram of mass M:

Balancing the vertical forces on M, we get,
Balancing the horizontal forces on M gives,
Substituting (2),
Substituting (1),
Hence we get the acceleration of the block of mass M as,
Note:
While drawing the free body diagrams, make sure that you don’t miss any of the forces acting on the masses. While marking the accelerations on the bodies keep in mind that you are dealing with a string constraint system. Also, chances are that you may miss normal forces while substituting, so take care of that too.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




