
Find the additive and multiplicative inverse of $ - \;\dfrac{8}{3}$
Answer
458.4k+ views
Hint:We have to find additive and multiplicative inverse of the given number. We know that two numbers are additive inverses if their sum is equals to zero whereas; two numbers are multiplicative inverses when their product is equals to one. Using the definitions we solve the problem.
Complete solution step by step:
Firstly we write down the number of which we want to find the additive and multiplicative inverses i.e.
$ - \;\dfrac{8}{3}$
We will find the additive inverse first using its definition i.e. two numbers are additive inverses if the sum of two is equals to zero. So we let a number to be $a$ and translating the definition into mathematical expression we have -
$ - \dfrac{8}{3} + a = 0$
Now we simply have to solve this algebraic equation to find the additive inverse so we transfer the constant part to the RHS of the equation to get -
$ \Rightarrow a = \dfrac{8}{3}$
This is the additive inverse of the given number.
Now we try to find the multiplicative inverse of the given number using its definition i.e. two numbers are multiplicative inverses if the product of two is equals to one. So we take number say $b$ and translate the definition into algebraic expression i.e.
$ - \dfrac{8}{3} \times b = 1$
Divide the above equation by $ - \dfrac{8}{3}$ we have
$b = - \dfrac{3}{8}$
This is the multiplicative inverse of the given number.
Hence we have solve both additive and multiplicative inverses of the number $ - \dfrac{8}{3}$
Note: While solving the question we saw that multiplicative inverse of a number is nothing but the reciprocal of the number and we could also solve it using cross multiplication method where we multiply components of a fraction upside-down in the other part of the equation like shown in the below figure –
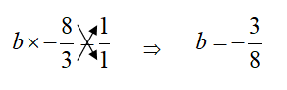
Complete solution step by step:
Firstly we write down the number of which we want to find the additive and multiplicative inverses i.e.
$ - \;\dfrac{8}{3}$
We will find the additive inverse first using its definition i.e. two numbers are additive inverses if the sum of two is equals to zero. So we let a number to be $a$ and translating the definition into mathematical expression we have -
$ - \dfrac{8}{3} + a = 0$
Now we simply have to solve this algebraic equation to find the additive inverse so we transfer the constant part to the RHS of the equation to get -
$ \Rightarrow a = \dfrac{8}{3}$
This is the additive inverse of the given number.
Now we try to find the multiplicative inverse of the given number using its definition i.e. two numbers are multiplicative inverses if the product of two is equals to one. So we take number say $b$ and translate the definition into algebraic expression i.e.
$ - \dfrac{8}{3} \times b = 1$
Divide the above equation by $ - \dfrac{8}{3}$ we have
$b = - \dfrac{3}{8}$
This is the multiplicative inverse of the given number.
Hence we have solve both additive and multiplicative inverses of the number $ - \dfrac{8}{3}$
Note: While solving the question we saw that multiplicative inverse of a number is nothing but the reciprocal of the number and we could also solve it using cross multiplication method where we multiply components of a fraction upside-down in the other part of the equation like shown in the below figure –
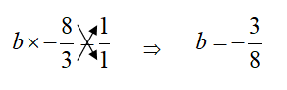
Recently Updated Pages
The correct geometry and hybridization for XeF4 are class 11 chemistry CBSE

Water softening by Clarks process uses ACalcium bicarbonate class 11 chemistry CBSE

With reference to graphite and diamond which of the class 11 chemistry CBSE

A certain household has consumed 250 units of energy class 11 physics CBSE

The lightest metal known is A beryllium B lithium C class 11 chemistry CBSE

What is the formula mass of the iodine molecule class 11 chemistry CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

The concept of the poverty line was first given in class 7 social science CBSE

Choose the correct option to fill in the blank with class 7 english CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

What crosssections do you get when you give a Vertical class 7 maths CBSE





