Question

Find the angle of intersection of two circles?
\[{{x}^{2}}+{{y}^{2}}+2gx+2fy+c=0\]
\[{{x}^{2}}+{{y}^{2}}+2{{g}_{1}}x+2{{f}_{1}}y+{{c}_{1}}=0\]

Answer 1

Hint: To solve this question, we will, first of all, compute the centre and radius of both the circles using the formula \[c=\left( \dfrac{-g}{a},\dfrac{-f}{a} \right)\] and \[r=\dfrac{1}{a}\sqrt{{{g}^{2}}+{{f}^{2}}-c}\] and then we will calculate the distance between \[{{c}_{1}}\] and \[{{c}_{2}}\] by using the distance between two points formula, \[d=\sqrt{{{\left( {{x}_{1}}-{{x}_{2}} \right)}^{2}}+{{\left( {{y}_{1}}-{{y}_{2}} \right)}^{2}}}.\] Finally, we will use the formula of cos of the angle in a triangle to get the result.

Complete step-by-step solution:
We are given the two circles below.
\[{{x}^{2}}+{{y}^{2}}+2gx+2fy+c=0.....\left( i \right)\]
\[{{x}^{2}}+{{y}^{2}}+2{{g}_{1}}x+2{{f}_{1}}y+{{c}_{1}}=0.....\left( ii \right)\]
They are given as

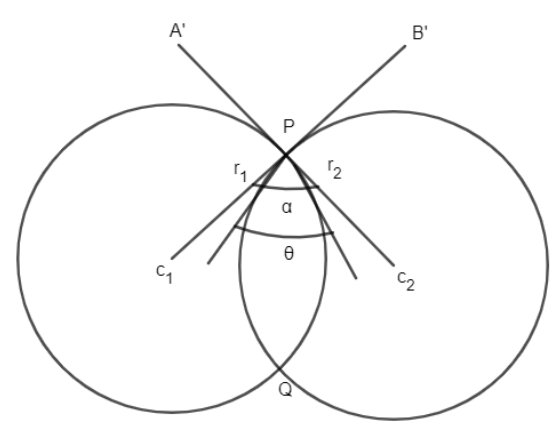
If the circles intersect at P, then the angle \[\theta \] is the angle between the tangents to both the circles at the point P. \[{{c}_{1}}\] and \[{{c}_{2}}\] are the centres of the circles given by the equation (i) and (ii) respectively. And the standard equation of a circle is of the form
\[a{{x}^{2}}+b{{y}^{2}}+2gx+2fy+2hxy+c=0\]
where a, b, h, g, f and c are constants and the radius of the circle is given by
\[r=\dfrac{1}{a}\sqrt{{{g}^{2}}+{{f}^{2}}-ca}\]
And the centre of the circle is given by
\[c=\left( \dfrac{-g}{a},\dfrac{-f}{a} \right)\]
Comparing this theory with our given equation (i) and equation (ii) of the circle, we have the centre as
\[{{c}_{1}}=\left( -g,-f \right)\]
\[{{c}_{2}}=\left( -{{g}_{1}},-{{f}_{1}} \right)\]
And radius is given as,
\[{{r}_{1}}=\sqrt{{{g}^{2}}+{{f}^{2}}-c}\]
\[{{r}_{2}}=\sqrt{{{g}_{1}}^{2}+{{f}_{1}}^{2}-{{c}_{1}}}\]
Clearly, as visible by the above diagram, the distance ‘d’ between the circles is given by
\[d=\left| {{c}_{1}}{{c}_{2}} \right|\]
The formula of the distance between the two points \[\left( {{x}_{1}},{{y}_{1}} \right)\] and \[\left( {{x}_{2}},{{y}_{2}} \right)\] is given by
\[d=\sqrt{{{\left( {{x}_{1}}-{{x}_{2}} \right)}^{2}}+{{\left( {{y}_{1}}-{{y}_{2}} \right)}^{2}}}\]
Using this formula to find the distance \[d=\left| {{c}_{1}}{{c}_{2}} \right|\] we have,
\[d=\sqrt{{{\left( g-{{g}_{1}} \right)}^{2}}+{{\left( f-{{f}_{1}} \right)}^{2}}}\]
\[\Rightarrow d=\sqrt{{{g}^{2}}+{{g}_{1}}^{2}-2g{{g}_{1}}+{{f}^{2}}+{{f}_{1}}^{2}-2f{{f}_{1}}}\]
\[\Rightarrow d=\sqrt{{{g}^{2}}+{{f}^{2}}+{{g}_{1}}^{2}+{{f}_{1}}^{2}+-2g{{g}_{1}}-2f{{f}_{1}}}\]
Now considering the triangle \[{{c}_{1}}P{{c}_{2}}\] and the angle \[\alpha \] between \[{{c}_{1}}P{{c}_{2}}.\]

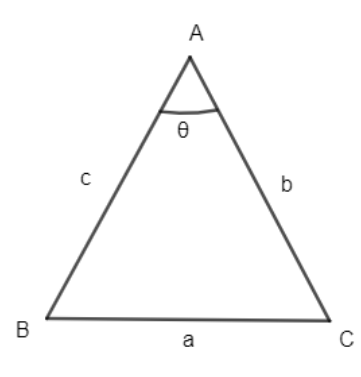
Now, if triangle ABC is given as below, where the angle \[\theta \] is \[\angle BAC,\] then if AB = c, AC = b and BC = a, then \[\cos \theta \] is given by the formula \[\cos \theta =\dfrac{{{b}^{2}}+{{c}^{2}}-{{a}^{2}}}{2bc}.\]
Applying this formula on the angle \[\alpha \] is given by
\[\cos \alpha =\dfrac{{{r}_{1}}^{2}+{{r}_{2}}^{2}-{{d}^{2}}}{2{{r}_{1}}{{r}_{2}}}\]
where \[\alpha \] is the angle \[\angle {{c}_{1}}P{{c}_{2}}.\]
Now, \[\theta \] is the angle \[\angle {{A}^{'}}P{{B}^{'}},\] this is so as vertically opposite angles are equal and \[\angle TPR=\theta .\]
\[\Rightarrow \angle {{A}^{'}}P{{B}^{'}}=\angle TPR=\theta \left[ \text{vertically opposite angles} \right]\]
Now, because \[{{A}^{'}}{{c}_{2}}\] and \[{{B}^{'}}{{c}_{1}}\] are tangles to the circles of the centre \[{{c}_{1}}\] and centre \[{{c}_{2}}\] respectively, then \[\angle {{B}^{'}}P{{c}_{2}}=\angle {{A}^{'}}P{{c}_{1}}={{90}^{\circ }}.\]
Finally, we have a full circle angle that is \[{{360}^{\circ }}.\]
\[\Rightarrow \angle {{B}^{'}}P{{c}_{2}}+\angle {{A}^{'}}P{{c}_{1}}+\angle {{A}^{'}}P{{B}^{'}}+\angle {{c}_{1}}P{{c}_{2}}={{360}^{\circ }}\]
\[\Rightarrow {{90}^{\circ }}+{{90}^{\circ }}+\alpha +\theta ={{360}^{\circ }}\]
\[\Rightarrow \alpha ={{180}^{\circ }}-\theta \]
Now, as \[\cos \alpha =\dfrac{{{r}_{1}}^{2}+{{r}_{2}}^{2}-{{d}^{2}}}{2{{r}_{1}}{{r}_{2}}}\]
\[\Rightarrow \cos \left( {{180}^{\circ }}-\theta \right)=\dfrac{{{r}_{1}}^{2}+{{r}_{2}}^{2}-{{d}^{2}}}{2{{r}_{1}}{{r}_{2}}}\]
Hence, the angle between the circle is given by \[\cos \left( {{180}^{\circ }}-\theta \right)=\dfrac{{{r}_{1}}^{2}+{{r}_{2}}^{2}-{{d}^{2}}}{2{{r}_{1}}{{r}_{2}}}\] where \[{{r}_{1}}\] is the radius of the circle \[{{x}^{2}}+{{y}^{2}}+2gx+2fy+c=0\] and \[{{r}_{2}}\] is the radius of the circle having equation \[{{x}^{2}}+{{y}^{2}}+2{{g}_{1}}x+2{{f}_{1}}y+{{c}_{1}}=0\] and d is the distance between the centre \[{{c}_{1}}\] and \[{{c}_{2}}\] of both the circles.

Note: Because \[\alpha \] is the angle between the centre \[{{c}_{1}}\] of the circle \[{{x}^{2}}+{{y}^{2}}+2gx+2fy+c=0\] and the centre \[{{c}_{2}}\] of the circle \[{{x}^{2}}+{{y}^{2}}+2{{g}_{1}}x+2{{f}_{1}}y+{{c}_{1}}=0.\] Therefore, we needed to calculate the value of \[\alpha .\] This \[\alpha \] will give the angle between two given circles. Also, in such cases always the angle from the centre of the two circles is measured.