
Find the area bounded by the ellipse
Answer
535.2k+ views
Hint: Simplify the given ellipse equation and integrate within the given ordinate limits to find the area.
An ellipse of the form
The ordinates given are
The shaded area is the area bounded by the ellipse and the given ordinates.
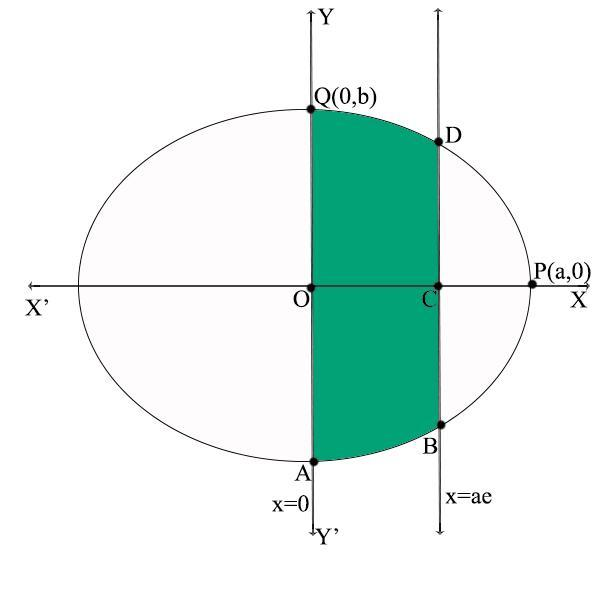
Required area = Area of the shaded region
=
=
The given equation is
Since, the area in equation (1) which is the area of QOCD is in the 1st quadrant. Hence, the value of y will be positive.
Hence,
Substituting (2) in (1),
Required area =
We know that,
Using this in the previous step, we get
Required area =
Required Area bounded by the ellipse
Note: The required area can also be found by integrating the entire shaded area QOABCD instead of finding
An ellipse of the form
The ordinates given are
The shaded area is the area bounded by the ellipse and the given ordinates.

Required area = Area of the shaded region
=
=
The given equation is
Since, the area in equation (1) which is the area of QOCD is in the 1st quadrant. Hence, the value of y will be positive.
Hence,
Substituting (2) in (1),
Required area =
We know that,
Using this in the previous step, we get
Required area =
Required Area bounded by the ellipse
Note: The required area can also be found by integrating the entire shaded area QOABCD instead of finding
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




