
How do you find the area of a circle using integration?
Answer
477.3k+ views
Hint: We use the polar coordinate system and write the area of the circle using integration of the small part of the circle which forms a triangle. Use limits of integration for angles as 0 to
* Area of a triangle is given by half of the base multiplied by its height.
Complete step-by-step answer:
Let us assume a circle having polar coordinates
We draw the diagram for the circle where we draw the small part of the circle with a small angle and arc length such that it becomes a straight line.
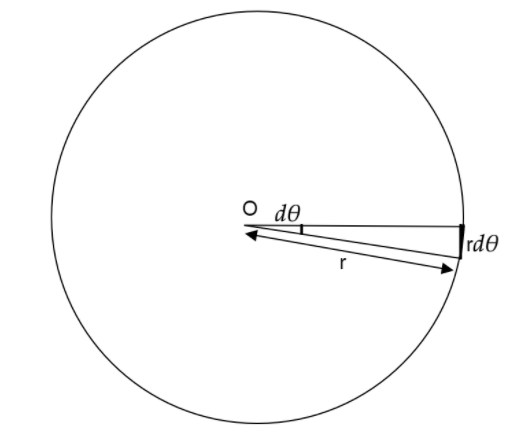
Then we take small part of the area which has radius
Since arc length of drawn portion can be given by
If we have to find the complete area of the circle then we will integrate the derivative of angle from 0 to
Now we bring out constant value from the integral
Now we integrate the angle from 0 to
Cancel same factors from numerator and denominator
Note:
Many students get confused between the polar coordinates as they are only aware about the equation of the circle in x-y coordinate. We have a polar form of coordinates where the coordinates are dependent on the angle and the radius of the circle. Also, when integrating the terms having double derivatives, always solve the integral inside the integral first and then apply the final integral to the calculated value.
* Area of a triangle is given by half of the base multiplied by its height.
Complete step-by-step answer:
Let us assume a circle having polar coordinates
We draw the diagram for the circle where we draw the small part of the circle with a small angle and arc length such that it becomes a straight line.

Then we take small part of the area which has radius
Since arc length of drawn portion can be given by
If we have to find the complete area of the circle then we will integrate the derivative of angle from 0 to
Now we bring out constant value from the integral
Now we integrate the angle from 0 to
Cancel same factors from numerator and denominator
Note:
Many students get confused between the polar coordinates as they are only aware about the equation of the circle in x-y coordinate. We have a polar form of coordinates where the coordinates are dependent on the angle and the radius of the circle. Also, when integrating the terms having double derivatives, always solve the integral inside the integral first and then apply the final integral to the calculated value.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




