
Answer
426.6k+ views
Hint: For answering this question we need to find the area of a quarter of a circle whose radius is $3cm$ . For doing that we will find the area of the circle and evaluate its quarter that is $\dfrac{1}{4}\left( Area \right)$ . The area of any circle with radius $r$ is given as $\pi {{r}^{2}}$ .
Complete step by step solution:
Now considering from the question we have been asked to find the area of a quarter of the given circle with radius $3cm$ .
From the basics of concept we know that quarter is ${{\dfrac{1}{4}}^{th}}$ part of the circle.
From the basics of concept we know that the area of any circle with radius $r$ is given as $\pi {{r}^{2}}$
Hence the area of a quarter of a circle will be given as $\dfrac{1}{4}\left( Area \right)=\dfrac{1}{4}\pi {{r}^{2}}$ .
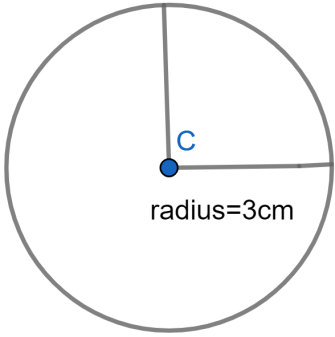
By applying this formula we will have $\dfrac{1}{4}\pi {{r}^{2}}=\dfrac{1}{4}\pi \left( 9 \right)=7.07c{{m}^{2}}$
Therefore we can conclude that the area of a quarter of a circle with radius $3cm$ is $7.07c{{m}^{2}}$ .
Note: During the process of solution of questions of this type we should be sure with the concepts we apply and the calculations we make. This is very simple and can be answered in a short span of time and very few mistakes are possible in it. Similarly the area of the full circle is given as $\pi {{r}^{2}}=\pi \left( 9 \right)=28.28c{{m}^{2}}$ . Similarly we can find the area of any circle or quarter. Area of a sector is given as $\dfrac{\theta }{{{360}^{\circ }}}\times \pi {{r}^{2}}$ .
Complete step by step solution:
Now considering from the question we have been asked to find the area of a quarter of the given circle with radius $3cm$ .
From the basics of concept we know that quarter is ${{\dfrac{1}{4}}^{th}}$ part of the circle.
From the basics of concept we know that the area of any circle with radius $r$ is given as $\pi {{r}^{2}}$
Hence the area of a quarter of a circle will be given as $\dfrac{1}{4}\left( Area \right)=\dfrac{1}{4}\pi {{r}^{2}}$ .

By applying this formula we will have $\dfrac{1}{4}\pi {{r}^{2}}=\dfrac{1}{4}\pi \left( 9 \right)=7.07c{{m}^{2}}$
Therefore we can conclude that the area of a quarter of a circle with radius $3cm$ is $7.07c{{m}^{2}}$ .
Note: During the process of solution of questions of this type we should be sure with the concepts we apply and the calculations we make. This is very simple and can be answered in a short span of time and very few mistakes are possible in it. Similarly the area of the full circle is given as $\pi {{r}^{2}}=\pi \left( 9 \right)=28.28c{{m}^{2}}$ . Similarly we can find the area of any circle or quarter. Area of a sector is given as $\dfrac{\theta }{{{360}^{\circ }}}\times \pi {{r}^{2}}$ .



