
Find the area of a right-angled triangle, the radius of whose circumcircle measures
Answer
495k+ views
Hint: Here, we will use the formula for finding the area of a right-angled triangle as shown below:
Complete step by step answer:
Step (1): First of all, we will draw the diagram according to the given information in the question where
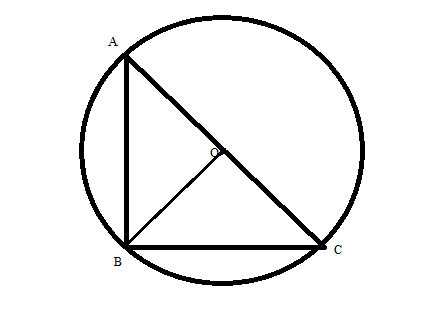
Where,
Step 2: Now we will draw a perpendicular from the point
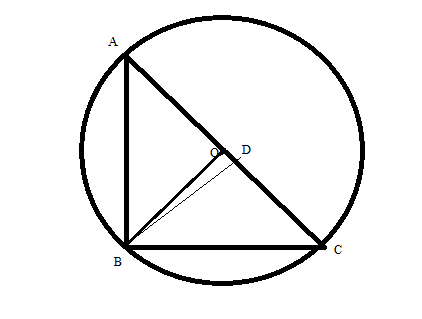
So,
Step 3: As we know that the radius of the circle is equal so,
By substituting the values of the radius
Step 4: Now, in the right-angled triangle
By solving the RHS side of the above expression (1), we get:
The area of the triangle is
Note:
Students needs to remember that the height circumcenter of a right-angle triangle is the midpoint of its hypotenuse:
In these questions, for finding the area of the triangle, we need to consider hypotenuse as a base and the altitude to the hypotenuse as height.
Complete step by step answer:
Step (1): First of all, we will draw the diagram according to the given information in the question where

Where,
Step 2: Now we will draw a perpendicular from the point

So,
Step 3: As we know that the radius of the circle is equal so,
By substituting the values of the radius
Step 4: Now, in the right-angled triangle
By solving the RHS side of the above expression (1), we get:
The area of the triangle is
Note:
Students needs to remember that the height circumcenter of a right-angle triangle is the midpoint of its hypotenuse:
In these questions, for finding the area of the triangle, we need to consider hypotenuse as a base and the altitude to the hypotenuse as height.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Discuss the main reasons for poverty in India




