
Find the area of pentagon ABCDE in which
Answer
424.8k+ views
1 likes
Hint: In this problem, we have to find the area of the pentagon as we are given
Complete step by step solution:
Here, we have to find the area of pentagon, where we are given
We can now draw the diagram and mark the length.
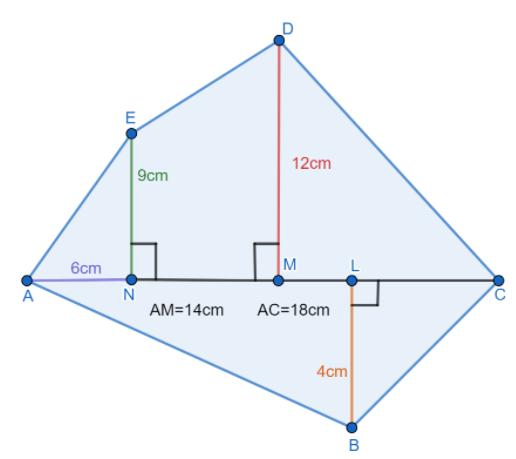
We can now find the area of each shape in the diagram and add them to find the area of the pentagon ABCDE.
We know that area of triangle formula is
We can first find the area of triangle ANE as AN = 6cm and NE = 9cm.
Area of triangle ANE = 27sq.cm ……. (1)
We can now find the area of ACB as AC= 18cm and AB = 4cm.
Area of triangle ACB = 36sq.cm …….. (2)
We can now find the area of triangle DMC as MD = 12 and
We can now substitute the length and height, we get
Area of triangle ACB = 24sq.cm ……… (3)
We can now find the area of trapezium using the formula ,
We can see in the diagram that bases are 9cm and 12 cm and
We can now substitute these values in area formula, we get
Area of trapezium = 84sq.cm …… (4)
We can now add (1), (2), (3), (4), we get
Area of the pentagon ABCDE,
Therefore, the area of the pentagon ABCDE is 171sq.cm.
Note: We should always remember that the area of the triangle formula is
Complete step by step solution:
Here, we have to find the area of pentagon, where we are given
We can now draw the diagram and mark the length.

We can now find the area of each shape in the diagram and add them to find the area of the pentagon ABCDE.
We know that area of triangle formula is
We can first find the area of triangle ANE as AN = 6cm and NE = 9cm.
Area of triangle ANE = 27sq.cm ……. (1)
We can now find the area of ACB as AC= 18cm and AB = 4cm.
Area of triangle ACB = 36sq.cm …….. (2)
We can now find the area of triangle DMC as MD = 12 and
We can now substitute the length and height, we get
Area of triangle ACB = 24sq.cm ……… (3)
We can now find the area of trapezium using the formula ,
We can see in the diagram that bases are 9cm and 12 cm and
We can now substitute these values in area formula, we get
Area of trapezium = 84sq.cm …… (4)
We can now add (1), (2), (3), (4), we get
Area of the pentagon ABCDE,
Therefore, the area of the pentagon ABCDE is 171sq.cm.
Note: We should always remember that the area of the triangle formula is
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




