
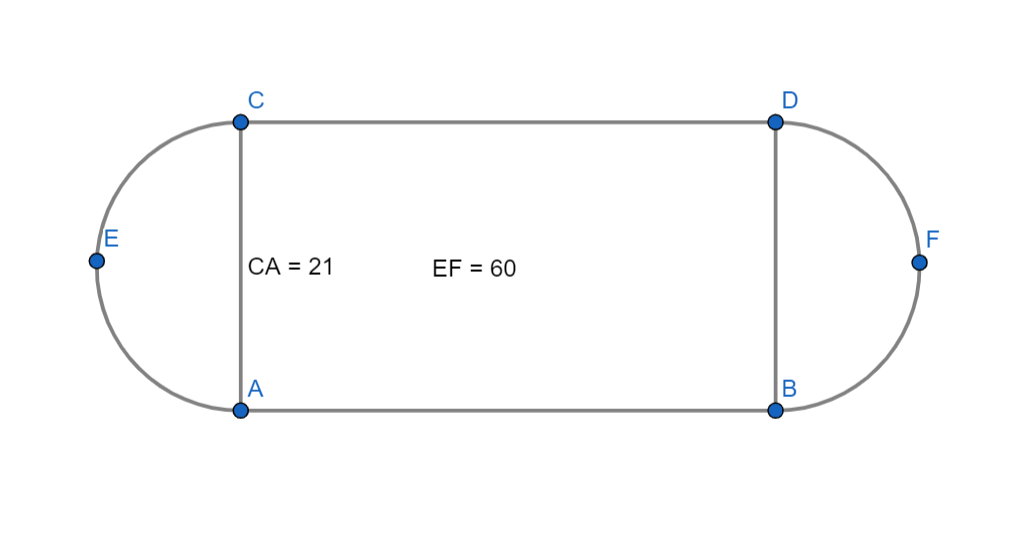
Find the area of the athletic ground given below. (All dimensions are in Meters)
A.
B.
C.
D. None

Answer
480.3k+ views
Hint: We can see two geometrical shapes in the given diagram. They are one rectangle and two semi circles. We have the width of the rectangle and total length of the ground which includes the radius of the two semi circles. So, to calculate the length of the rectangle we will subtract the radiuses of two semi circles from the total length of ground. Now we will calculate the area of each shape and sum up them to get the required result.
Complete step-by-step answer:
Given that, The athletic ground is given below
The diameter of the two semi circles is
Now the area of the one semi circle is given by
Now the area of the two semi circles is given by
In the problem we have the width of the rectangle as
So the area of the rectangle is calculated as
Now the total area of the ground is equal to the sum of the area of the rectangle and the total areas of the two semi circles.
So, the correct answer is “Option B”.
Note: We can directly use the below formula to get the area of the athletic ground.
From both the methods we got the same result.
Complete step-by-step answer:
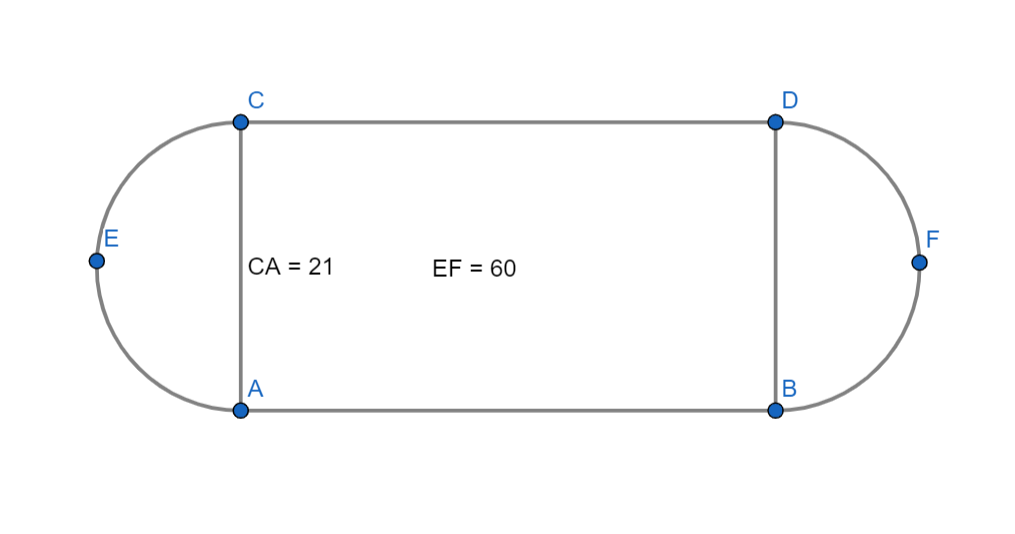
Given that, The athletic ground is given below

The diameter of the two semi circles is
Now the area of the one semi circle is given by
Now the area of the two semi circles is given by
In the problem we have the width of the rectangle as
So the area of the rectangle is calculated as
Now the total area of the ground is equal to the sum of the area of the rectangle and the total areas of the two semi circles.
So, the correct answer is “Option B”.
Note: We can directly use the below formula to get the area of the athletic ground.
From both the methods we got the same result.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE




