
Find the area of the shaded region.
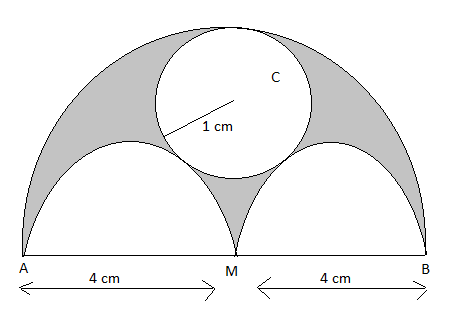

Answer
512.4k+ views
1 likes
Hint: To find the area of the shaded region in the given figure we will subtract the area of the circle having radius 1 cm and the two semi-circle having radius 2 cm from the bigger semi-circle of radius 4 cm. Then we will get the area of the shaded region.
Complete step-by-step answer:
We have been given the figure as follows:
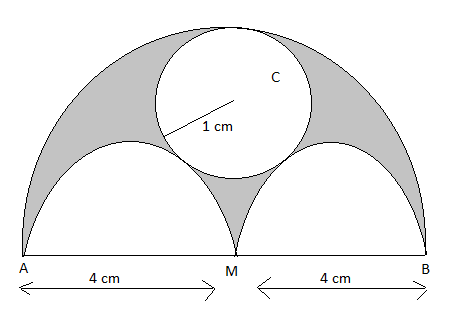
From the given figure, we can say that the area of the shaded region is equal to the area obtained by subtracting the area of the circle with C as center and the two semi-circles with AM and MB as diameter from the bigger semi-circle having AB as diameter.
We know that area of a circle having D as diameter is as follows:
Area
So, area of semi-circle
Now the area of semi-circle having AM = 4 cm as diameter
Area of semi-circle having MB = 4 cm as diameter
Area of circle having C as center and diameter
Area of semicircle having AB = 8 cm as diameter
So the area of the shaded region = area of semi-circle having AB as diameter – (area of the circle having C as center + 2 area of semi-circle with AM as diameter)
Also,
Area of shaded region
Therefore, the area of the shaded region is
Note: Be careful while calculating the area as we have been given the diameter of some circle and radius of a circle so accordingly use the formula to calculate the area.
Complete step-by-step answer:
We have been given the figure as follows:

From the given figure, we can say that the area of the shaded region is equal to the area obtained by subtracting the area of the circle with C as center and the two semi-circles with AM and MB as diameter from the bigger semi-circle having AB as diameter.
We know that area of a circle having D as diameter is as follows:
Area
So, area of semi-circle
Now the area of semi-circle having AM = 4 cm as diameter
Area of semi-circle having MB = 4 cm as diameter
Area of circle having C as center and diameter
Area of semicircle having AB = 8 cm as diameter
So the area of the shaded region = area of semi-circle having AB as diameter – (area of the circle having C as center + 2 area of semi-circle with AM as diameter)
Also,
Area of shaded region
Therefore, the area of the shaded region is
Note: Be careful while calculating the area as we have been given the diameter of some circle and radius of a circle so accordingly use the formula to calculate the area.
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Discuss the main reasons for poverty in India

How fast is 60 miles per hour in kilometres per ho class 10 maths CBSE

Choose the feminine form of the given noun Fox AFoxess class 10 english CBSE

Write examples of herbivores carnivores and omnivo class 10 biology CBSE




