
Find the area of the triangle whose vertices are (4,5), (0,7), (-1,1).
Answer
493.2k+ views
Hint: In order to solve this problem, we need to use the formula of area of a triangle using the vertices of the triangle. The formula for the area of the triangle is given by
Complete step-by-step answer:
We need to find the area of the triangle. For the area of the triangle, we need to find the sides of the triangles.
For a better interpretation, we need to draw a rough sketch of the triangle.
The figure is as below,
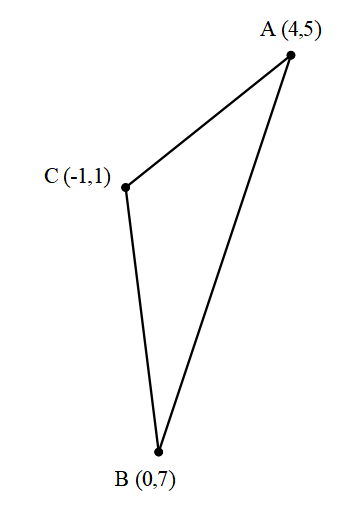
Let the triangle be ABC with vertices A (4,5) , B (0,7) , C (-1,1).
Let, the coordinates of A be
Let, the coordinates of B be
Let, the coordinates of C be
The area of the triangle
Where,
We can substitute the values and find the answer to the area of the triangle.
Substituting we get,
Simplifying further we get,
Therefore, the area of the triangle is 13 square units.
Note: We need to note that we can start naming coordinates from any coordinates the answer we get will be the same. Even if we get the answer negative we only need to take the positive values because the area cannot be negative ever. Also, as we have not given the units we will assume the units to be square units.
Complete step-by-step answer:
We need to find the area of the triangle. For the area of the triangle, we need to find the sides of the triangles.
For a better interpretation, we need to draw a rough sketch of the triangle.
The figure is as below,

Let the triangle be ABC with vertices A (4,5) , B (0,7) , C (-1,1).
Let, the coordinates of A be
Let, the coordinates of B be
Let, the coordinates of C be
The area of the triangle
Where,
We can substitute the values and find the answer to the area of the triangle.
Substituting we get,
Simplifying further we get,
Therefore, the area of the triangle is 13 square units.
Note: We need to note that we can start naming coordinates from any coordinates the answer we get will be the same. Even if we get the answer negative we only need to take the positive values because the area cannot be negative ever. Also, as we have not given the units we will assume the units to be square units.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




