Question

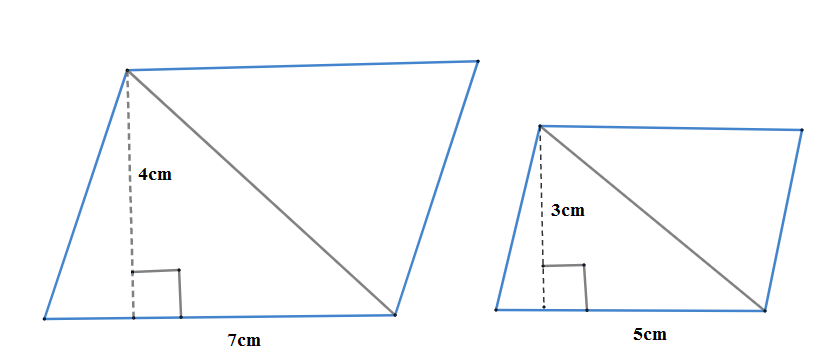
Find the areas of following parallelograms. \[\]

Answer 1

Hint: We recall the definition of parallelogram, the height $h$ of which is length perpendicular dropped from one vertex to opposite side, the base $b$which is the length of the opposite side where foot of the perpendicular lie. We recall the area of the parallelogram as a product of height and base $bh$. We use the length of height and length of base in the given parallelograms and use the formula to get the area. \[\]

Complete step by step answer:

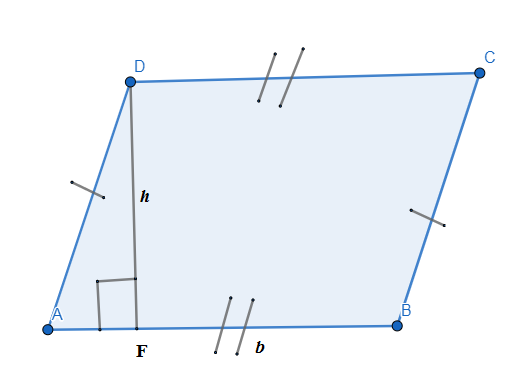
We know that a parallelogram is a quadrilateral where the opposite sides are equal in length and parallel to each other. We have drawn the figure of the parallelogram ABCD where AB=CD and AD=BC. The parallelogram also has equal opposite angles. If draw any perpendicular from any vertex on the opposite side, the perpendicular is called height of the parallelogram conventionally denoted as $h$ and the where the opposite side is called base denoted as $b.$ In the figure the perpendicular is drawn from vertex D on AB. So $h=DF,b=AB$. \[\]
We also know that the area of the parallelogram is the product of the lengths of base and height. So the area $A$ of the of the parallelogram can be written as
\[A=DF\times AB=h\times b=bh\]
Let us observe the first parallelogram. \[\]
 

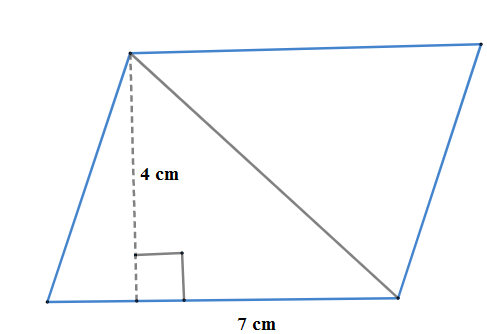
The dotted line is perpendicular or height of the parallelogram and length of the height is given as $h=4$cm. The length of the base is given as $b=7$cm. So the area $A$ of the parallelogram is
\[A=h\times b=4\text{ cm}\times 7\text{ cm}=28\text{ c}{{\text{m}}^{2}}\]
Let us observe the second parallelogram. \[\]
.

Here we are given the length of the height $h=3$cm and length of the base $b=5$cm. So the area of the parallelogram is,
\[A=h\times b=3\text{ cm}\times 5\text{ cm}=15\text{ c}{{\text{m}}^{2}}\]

Note: We note that $\text{c}{{\text{m}}^{2}}$ (read as centimetre squared or square centimeter) is a unit of area obtained from multiplying cm with cm. It is not necessary that the height of the parallelogram will lie in the interior of the parallelogram, for example in our figure if we drop perpendicular from C on AB it will lie outside the parallelogram ABCD.