
Answer
405k+ views
Hint: First we need to define the coordinates for the given masses. The centre of mass of the system of given masses can be calculated by taking the ratio of sum of products of the masses and their distances from the origin to the sum of the given masses.
Formula used:
The centre of mass of a system of mass can be given as
$C = \dfrac{{\sum {{m_i}r_i^2} }}{{\sum {{m_i}} }}$
Here ${m_i}$ represents the mass of the ith particle while ${r_i}$ represents the distance of the ith mass from the origin.
Detailed step by step solution:
The centre of mass of a system of masses is defined as the point on a body about which the whole mass of the given system is concentrated. Mathematically, it is equal to the ratio of sum of products of the masses and their distances from the origin to the sum of the given masses.
We are given three particles whose masses are given as
$\begin{array}{l}
{m_1} = 100g\\
{m_2} = 150g\\
{m_3} = 200g
\end{array}$
These three particles are arranged on the vertices of an equilateral triangle. Each side of the equilateral triangle has length of 0.5 m. We can draw the following diagram for this arrangement.
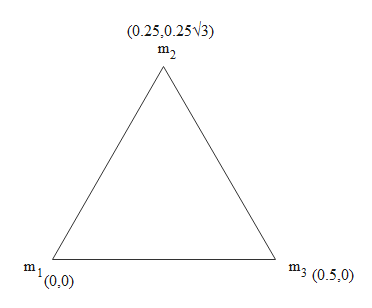
Now based on this diagram, we have three masses and their coordinates. Now we can find the coordinates of the centre of mass by using the formula given in equation (i). First, let us calculate the x-coordinate of the centre of mass. For this we will use the x-coordinates of the given masses. This is done in the following way.
$\begin{array}{l}
\left( {{x_1},{y_1}} \right) = \left( {0,0} \right)\\
\left( {{x_2},{y_2}} \right) = \left( {0.5,0} \right)\\
\left( {{x_3},{y_3}} \right) = \left( {0.25,0.25\sqrt 3 } \right)\\
{x_{CM}} = \dfrac{{{m_1}{x_1} + {m_2}{x_2} + {m_3}{x_3}}}{{{m_1} + {m_2}\, + {m_3}}}\\
= \dfrac{{\left( {100 \times 0} \right) + \left( {150 \times 0.5} \right) + \left( {200 \times 0.25} \right)}}{{\left( {100 + 150 + 200} \right)}}\\
\therefore {x_{CM}} = 0.278
\end{array}$
Similarly, we need to calculate the y-coordinate also which can be calculated in the following.
$\begin{array}{l}
{y_{CM}} = \dfrac{{{m_1}{y_1} + {m_2}{y_2} + {m_3}{y_3}}}{{{m_1} + {m_2}\, + {m_3}}}\\
= \dfrac{{\left( {100 \times 0} \right) + \left( {150 \times 0} \right) + \left( {200 \times 0.25\sqrt 3 } \right)}}{{\left( {100 + 150 + 200} \right)}}\\
\therefore {y_{CM}} = 0.192
\end{array}$
Hence, the coordinates of the centre of mass are given as $\left( {0.278,0.192} \right)$.
Note: We have derived the coordinates of the various masses by using simple rules of Pythagoras theorem. We take the first mass at origin and derive the coordinates of the rest of the particles based on given information and properties of the equilateral triangle.
Formula used:
The centre of mass of a system of mass can be given as
$C = \dfrac{{\sum {{m_i}r_i^2} }}{{\sum {{m_i}} }}$
Here ${m_i}$ represents the mass of the ith particle while ${r_i}$ represents the distance of the ith mass from the origin.
Detailed step by step solution:
The centre of mass of a system of masses is defined as the point on a body about which the whole mass of the given system is concentrated. Mathematically, it is equal to the ratio of sum of products of the masses and their distances from the origin to the sum of the given masses.
We are given three particles whose masses are given as
$\begin{array}{l}
{m_1} = 100g\\
{m_2} = 150g\\
{m_3} = 200g
\end{array}$
These three particles are arranged on the vertices of an equilateral triangle. Each side of the equilateral triangle has length of 0.5 m. We can draw the following diagram for this arrangement.
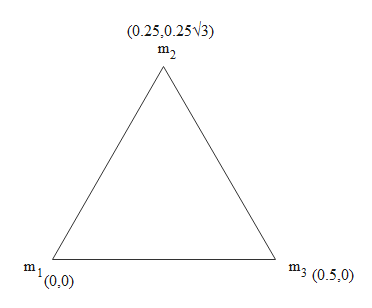
Now based on this diagram, we have three masses and their coordinates. Now we can find the coordinates of the centre of mass by using the formula given in equation (i). First, let us calculate the x-coordinate of the centre of mass. For this we will use the x-coordinates of the given masses. This is done in the following way.
$\begin{array}{l}
\left( {{x_1},{y_1}} \right) = \left( {0,0} \right)\\
\left( {{x_2},{y_2}} \right) = \left( {0.5,0} \right)\\
\left( {{x_3},{y_3}} \right) = \left( {0.25,0.25\sqrt 3 } \right)\\
{x_{CM}} = \dfrac{{{m_1}{x_1} + {m_2}{x_2} + {m_3}{x_3}}}{{{m_1} + {m_2}\, + {m_3}}}\\
= \dfrac{{\left( {100 \times 0} \right) + \left( {150 \times 0.5} \right) + \left( {200 \times 0.25} \right)}}{{\left( {100 + 150 + 200} \right)}}\\
\therefore {x_{CM}} = 0.278
\end{array}$
Similarly, we need to calculate the y-coordinate also which can be calculated in the following.
$\begin{array}{l}
{y_{CM}} = \dfrac{{{m_1}{y_1} + {m_2}{y_2} + {m_3}{y_3}}}{{{m_1} + {m_2}\, + {m_3}}}\\
= \dfrac{{\left( {100 \times 0} \right) + \left( {150 \times 0} \right) + \left( {200 \times 0.25\sqrt 3 } \right)}}{{\left( {100 + 150 + 200} \right)}}\\
\therefore {y_{CM}} = 0.192
\end{array}$
Hence, the coordinates of the centre of mass are given as $\left( {0.278,0.192} \right)$.
Note: We have derived the coordinates of the various masses by using simple rules of Pythagoras theorem. We take the first mass at origin and derive the coordinates of the rest of the particles based on given information and properties of the equilateral triangle.
Recently Updated Pages
10 Examples of Evaporation in Daily Life with Explanations

10 Examples of Diffusion in Everyday Life

1 g of dry green algae absorb 47 times 10 3 moles of class 11 chemistry CBSE

What is the meaning of celestial class 10 social science CBSE

What causes groundwater depletion How can it be re class 10 chemistry CBSE

Under which different types can the following changes class 10 physics CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Distinguish between the following Ferrous and nonferrous class 9 social science CBSE

The term ISWM refers to A Integrated Solid Waste Machine class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Which is the longest day and shortest night in the class 11 sst CBSE

In a democracy the final decisionmaking power rests class 11 social science CBSE




