Question

Find the diagonal of a square whose sides are 16 cm.

Answer 1

Hint: We are given that the side of the square is 16 cm and we have to find the value of the length of the diagonal of the square. To do this, we will learn about the square so that we can get the relation between the sides of the square and the diagonal. We will use Pythagoras theorem \[{{H}^{2}}={{P}^{2}}+{{B}^{2}}\] to solve our problem and last we will simplify and solve for the value of the diagonal.

Complete step by step answer:
We are asked to find the length of the diagonal of the square whose side is 16 cm in length. To find the length of the diameter we will have to learn about the square. So, we will start with the characteristic of the square. A square is one kind of quadrilateral in which all the sides are of equal length and the angle formed by the sides are 90 degrees each.

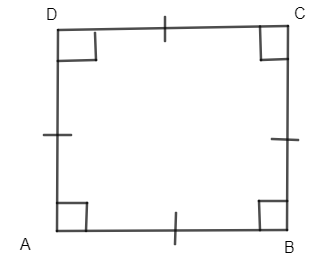
\[AB=BC=CD=DA.......\left( i \right)\]
Now, the diagonal of a square is the line that is drawn to join the opposite end of the vertex.

So, in the figure, AC and BD are the diagonals.
Now, as we learn that all the angles are of 90 degrees each, so we get, in square ABCD,

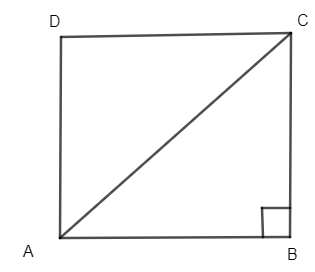
there is a triangle ABC in which angle B is 90 degrees, so triangle ABC is a right-angled triangle. So, as we know in a right-angled triangle, we can apply the Pythagoras theorem which says
\[{{H}^{2}}={{P}^{2}}+{{B}^{2}}.....\left( ii \right)\]
In triangle ABC, AC is the hypotenuse (H) as it is the opposite to 90-degree angle and BC and AB are perpendicular and base as in square, all sides are equal. So,
BC = AB [From (i)]
And the sides of the square are of length 16cm. So
\[BC=AB=16\]
Now using this in (ii), we get,
\[{{H}^{2}}={{P}^{2}}+{{B}^{2}}\]
\[\Rightarrow A{{C}^{2}}=A{{B}^{2}}+B{{C}^{2}}\]
\[\Rightarrow A{{C}^{2}}=B{{C}^{2}}+{{\left( BC \right)}^{2}}\left[ \text{As }AB=BC \right]\]
As the side of the square is 16cm, so we get,
\[\Rightarrow A{{C}^{2}}={{16}^{2}}+{{16}^{2}}\]
\[\Rightarrow A{{C}^{2}}=256+256\]
\[\Rightarrow A{{C}^{2}}=512\]
Now, simplifying, we get,
\[\Rightarrow AC=\sqrt{512}\]
We will factorize 512 to get the square root. So, we get,
\[AC=\sqrt{\left[ 2\times 2 \right]\times \left[ 2\times 2 \right]\times \left[ 2\times 2 \right]\times \left[ 2\times 2 \right]\times 2}\]
We will take the pairs out, we get,
\[AC=2\times 2\times 2\times 2\sqrt{2}\]
So, \[AC=16\sqrt{2}\]
So, we get the length of the diagonal as \[16\sqrt{2}cm.\]

Note:
Students need to remember that when we add 2 square values, the root of them is not the sum of the value that is \[\sqrt{{{16}^{2}}+{{16}^{2}}}\ne 16+16.\] Also, all the diagonal of the squares are of equal length. So, we can find any one of the two (AC or BD).