Question

Find the distance between the points (0, 5) and (-5, 0) is
A. $2\sqrt{5}$
B. $5\sqrt{2}$
C. 5
D. 10

Answer 1

Hint: To solve this question, we should know about a few concepts of coordinate geometry like distance formula, that is distance between two points $\left( {{x}_{1}},{{y}_{1}} \right)$ and $\left( {{x}_{2}},{{y}_{2}} \right)$ can be calculated using the formula, $\sqrt{{{\left( {{x}_{1}}-{{x}_{2}} \right)}^{2}}+{{\left( {{y}_{1}}-{{y}_{2}} \right)}^{2}}}$. Also, we need to take care of the signs while solving this question.

Complete step-by-step solution -
In this question, we have been given two points, that is, (0, 5) and (-5, 0). And we have been asked to find the distance between them. For that we require the knowledge about distance formula of coordinate geometry, which states that distance between any two given coordinates like $\left( {{x}_{1}},{{y}_{1}} \right)$ and $\left( {{x}_{2}},{{y}_{2}} \right)$ is given by $\sqrt{{{\left( {{x}_{1}}-{{x}_{2}} \right)}^{2}}+{{\left( {{y}_{1}}-{{y}_{2}} \right)}^{2}}}$. Now, we will consider ${{x}_{1}}=0,{{y}_{1}}=5$ and ${{x}_{2}}=-5,{{y}_{2}}=0$. We will put these values in the distance formula, we get the distance between the two points (0, 5) and (-5, 0) as,
$\sqrt{{{\left[ 0-\left( -5 \right) \right]}^{2}}+{{\left[ 5-0 \right]}^{2}}}$
Simplifying it further, we get,
$\begin{align}
  & \sqrt{{{\left[ 0+5 \right]}^{2}}+{{\left[ 5-0 \right]}^{2}}} \\
 & \Rightarrow \sqrt{{{\left( 5 \right)}^{2}}+{{\left( 5 \right)}^{2}}} \\
\end{align}$
Now, we know that ${{5}^{2}}=5\times 5=25$. So, we will get the distance between (0, 5) and (-5, 0) as, $=\sqrt{25+25}$
Now, we know that 25 +25 = 50, so we will get $\sqrt{50}$. We know that 50 can be expressed as $25\times 2$, where 25 can be represented as ${{5}^{2}}$. So, we will get, $\sqrt{{{5}^{2}}\times 2}$, which can be further written as, $\sqrt{{{5}^{2}}}\times \sqrt{2}$. We know that the square root of square of any term gives us the same term, so we get, $5\sqrt{2}$. Hence, we can say that the distance between the points (0, 5) and (-5, 0) is $5\sqrt{2}$.
Therefore, the correct answer is option B.

Note: We can also solve this question by plotting points on graph and then applying the Pythagoras theorem. Like the figure below,

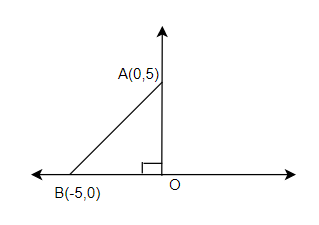
Now, we can see that triangle AOB is a right-angled triangle. So, by Pythagoras theorem, we can write, ${{\left( AO \right)}^{2}}+{{\left( OB \right)}^{2}}={{\left( AB \right)}^{2}}$ and we have AO = OB = 5. So, we will get ${{\left( 5 \right)}^{2}}+{{\left( 5 \right)}^{2}}={{\left( AB \right)}^{2}}$. And after simplifying this we will get, $AB=5\sqrt{2}$.