
Find the efficiency of the cycle.

Answer
495.6k+ views
Hint
To answer this question, we need to find the heat transfer and work done for all the three processes in the cycle. Then, we need to put these into the efficiency formula.
-
-
-
Complete step by step answer
We know that the ideal gas equation is given by
We know that
Dividing by
Now
Now, we calculate the temperatures at the three states given.
Applying (1) at the state 1, we get
Separating
Similarly, applying equation (1) on state 2 and state 3, we get
Now, for calculating the efficiency, we have to make the calculation for the work done and heat transfer for the three processes.
For process
We know that for an isothermal process, the work done is given by
Therefore work done for this process
Substituting the values of
On simplifying, we get
We know that
From the first law of thermodynamics, we have
We know that
The negative sign shows that heat is released in this process.
For process
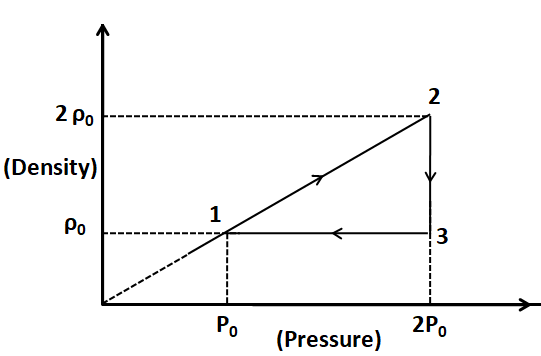
As we can see from the plot given, the process
We know that work done in an isobaric process is given by
From the ideal gas equation
Taking delta both the sides
For an isobaric process, we have
From (4), work done for an isobaric process
So, for the process
Putting the values of
On simplifying, we get
Now, we know that heat transfer in an isobaric process is given by
So, for the process
Simplifying, we get
For process
From the plot, we can see that the density
We know that the work done in an isochoric process is zero.
Also, the heat transfer in an isochoric process is given as
Putting the values of
The negative sign shows that heat is released in this process.
Now, the efficiency,
Total work done,
From (2), (5) and (7)
Total heat supplied,
From (6)
Simplifying, we get
We know that
Finally, we have
Note
We know that the efficiency cannot be more than or equal to one. It is always less than one. So, we should always check that the final expression of efficiency which we are getting should be less than unity. If it is more than unity, then this means that there is some mistake in the solution.
To answer this question, we need to find the heat transfer and work done for all the three processes in the cycle. Then, we need to put these into the efficiency formula.
-
-
-
Complete step by step answer
We know that the ideal gas equation is given by
We know that
Dividing by
Now
Now, we calculate the temperatures at the three states given.
Applying (1) at the state 1, we get
Separating
Similarly, applying equation (1) on state 2 and state 3, we get
Now, for calculating the efficiency, we have to make the calculation for the work done and heat transfer for the three processes.
For process
We know that for an isothermal process, the work done is given by
Therefore work done for this process
Substituting the values of
On simplifying, we get
We know that
From the first law of thermodynamics, we have
We know that
The negative sign shows that heat is released in this process.
For process
As we can see from the plot given, the process
We know that work done in an isobaric process is given by
From the ideal gas equation
Taking delta both the sides
For an isobaric process, we have
From (4), work done for an isobaric process
So, for the process
Putting the values of
On simplifying, we get
Now, we know that heat transfer in an isobaric process is given by
So, for the process
Simplifying, we get
For process
From the plot, we can see that the density
We know that the work done in an isochoric process is zero.
Also, the heat transfer in an isochoric process is given as
Putting the values of
The negative sign shows that heat is released in this process.
Now, the efficiency,
Total work done,
From (2), (5) and (7)
Total heat supplied,
From (6)
Simplifying, we get
We know that
Finally, we have
Note
We know that the efficiency cannot be more than or equal to one. It is always less than one. So, we should always check that the final expression of efficiency which we are getting should be less than unity. If it is more than unity, then this means that there is some mistake in the solution.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




