
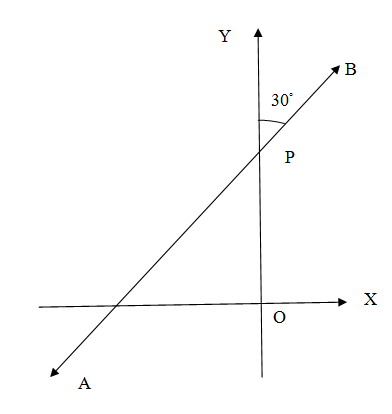
Find the equation of the line AB in the following figure, given

Answer
493.5k+ views
1 likes
Hint:We find the equation of the line AB in slope point form with slope
Complete step by step answer:
We see in the given figure that the starlight line AB is inclined on
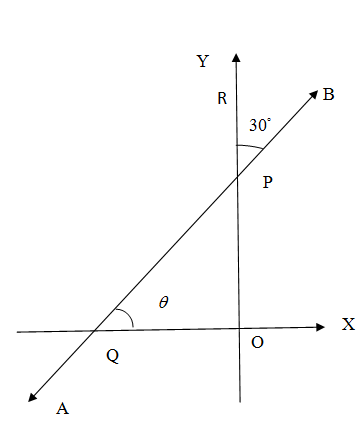
We know the
We have
We know that the slope of a line is tangent of the angle it makes with positive
We know the equation of the line in slope point form with slope
The slope of the line AB is
Note:
We can also find the equation of the any line with slope
Complete step by step answer:
We see in the given figure that the starlight line AB is inclined on

We know the
We have
We know that the slope of a line is tangent of the angle it makes with positive
We know the equation of the line in slope point form with slope
The slope of the line AB is
Note:
We can also find the equation of the any line with slope
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE




