
Find the equation of the parabola which is symmetric about the y-axis and passes through the point (3, -9).
Answer
477.9k+ views
Hint: We solve this by finding where the points (3, -9) lie in the equation of parabola. Since the parabola is symmetric about the y-axis is
Complete step-by-step answer:
We know the equation of parabola about the y-axis is
Since the points (3, -9) lie in the fourth quadrant so the equation of parabola about the y-axis is
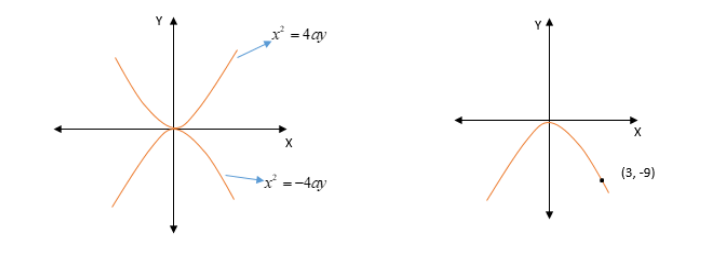
Hence, the equation of parabola is
We need to find the value of ‘a’.
Now parabola passes through (3, -9), put
We know the product of negative and negative is positive and rearranging the above equation we get:
We know the value of ‘a’. Now substituting in the equation (1). We get
Cancelling 4,
The equation of the parabola which is symmetric about the y-axis and passes through the point (3, -9) is
So, the correct answer is “
Note: If they ask the same question with the same points with a symmetric about the x-axis then we have the equation of parabola is
Complete step-by-step answer:
We know the equation of parabola about the y-axis is
Since the points (3, -9) lie in the fourth quadrant so the equation of parabola about the y-axis is

Hence, the equation of parabola is
We need to find the value of ‘a’.
Now parabola passes through (3, -9), put
We know the product of negative and negative is positive and rearranging the above equation we get:
We know the value of ‘a’. Now substituting in the equation (1). We get
Cancelling 4,
The equation of the parabola which is symmetric about the y-axis and passes through the point (3, -9) is
So, the correct answer is “
Note: If they ask the same question with the same points with a symmetric about the x-axis then we have the equation of parabola is
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




