
How do you find the factor tree of
Answer
473.4k+ views
Hint:To draw the factor tree of
Complete step by step solution:
A factor tree is an extraordinary graph where we discover the factors of a number and afterward factors of those numbers until we can factor them no more. Eventually, what we get are for the most part the prime factors of the first number.
The number
Factor Tree of
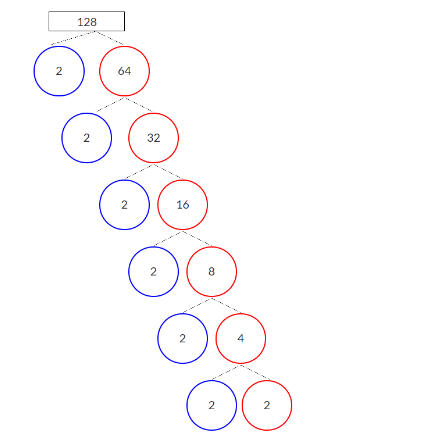
If we try to write
On parting
On parting
On parting
On parting
On parting
Out and out Expressing the number in the terms of only prime factors would be
Note: 1.Carefully factorise the number such that no factor can be made in the end .
2.Don’t forgot to cross-check your factor tree once to make sure that you have factored every level
properly.
Complete step by step solution:
A factor tree is an extraordinary graph where we discover the factors of a number and afterward factors of those numbers until we can factor them no more. Eventually, what we get are for the most part the prime factors of the first number.
The number
Factor Tree of

If we try to write
On parting
On parting
On parting
On parting
On parting
Out and out Expressing the number in the terms of only prime factors would be
Note: 1.Carefully factorise the number such that no factor can be made in the end .
2.Don’t forgot to cross-check your factor tree once to make sure that you have factored every level
properly.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




