Question

Find the least number which when divided by 25, 40 and 60 leaves 9 as the remainder in each case.

Answer 1

Hint: First of all let the least number be N. Then using the division theorem, N = dq + r, write, N = 25a + 9, N = 40b + 9 and N = 60c + 9. Find N by taking LCM of 25, 40 and 9 and adding 9 to it.

“Complete step-by-step answer:”
Here, we have to find the least number which when divided by 25, 40 and 60 leaves 9 as the remainder in each case.
Before solving this question, we must know what division theorem is. Division theorem states that “If ‘n’ is any integer and ‘d’ is a positive integer, there exist unique integers ‘q’ and ‘r’ such that,
$n=dq+r$ where 0${\leq}$r<$d$
Here, ‘n’ is the number or the dividend, ‘d’ is the divisor, ‘q’ is the quotient and ‘r’ is the remainder.
For example, if we divide a number or dividend that is, say 16 by divisor, say 5, we get quotient as 3 and remainder as 1.
By division theorem, we can write it as
16 = 5 (2) + 1
Now, we have to find the least number which when divided by 25, 40, 60 leaves 9 as the remainder in each case.
Here, let us consider the least number to be N. As we know that 25, 40, 60 are divisors and 9 is the remainder in each case. Therefore, by division therefore, we get
\[\begin{align}
  & N=25a+9....\left( i \right) \\
 & N=40b+9....\left( ii \right) \\
 & N=60c+9....\left( iii \right) \\
\end{align}\]
where a, b, and c are quotients in each case.
By subtracting 9 from both sides of the equation (i), (ii) and (iii), we get,
\[\begin{align}
  & \Rightarrow \left( N-9 \right)=25a \\
 & \Rightarrow \left( N-9 \right)=40b \\
 & \Rightarrow \left( N-9 \right)=60c \\
\end{align}\]
As we know that a, b, and c are integers, therefore we have to find the least value of (N – 9) such that it is a multiple of 25, 40 and 60. That means we have to find the LCM or lowest common multiple of 25, 40 and 60.
Now, we will find the LCM of 25, 40 and 60 as follows:

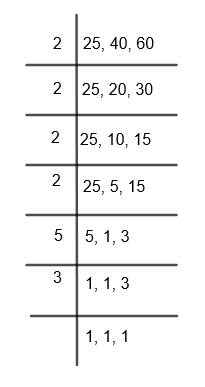
Therefore, we get LCM of 25, 40 and 60 = 2 x 2 x 2 x 5 x 5 x 3 = 600
Therefore, we get the least value of (N – 9) = 600.
Therefore, we get the least value of N = 609.
Hence, 609 is the least number which when divided by 25, 40 and 60 leaves 9 as remainder in each case.

Note: Here, students can cross-check by dividing 609 by 25, 40 and 60 and see if it is leaving remainder 9 or not. Also for these types of questions, students can directly use the formula that is
N = (LCM of divisors) + (Common Remainder (R))
Here, N is the least number which when divided by different divisors gives a common remainder R.