
Find the length of the transverse common tangent to two circle of radii 8 cm and 3 cm, the centres of the circles be at a distance 13 cm is
(a)
(b)
(c)
(d)
Answer
521.1k+ views
Hint:Find the length of the transverse common tangent to circle by using the properties:-
(i) Radius is perpendicular to tangent at the point of tangency
(ii) Radius of both the circles at the tangency point will be parallel to each other.
Length of common transverse tangent is given as
Complete step-by-step answer:
Here, we have two circles with radii 8 cm and 3 cm, and we need to determine the length of the transverse common tangent, if the distance between centres of both the circles is 13 cm.
So, length of transverse tangent can be calculated
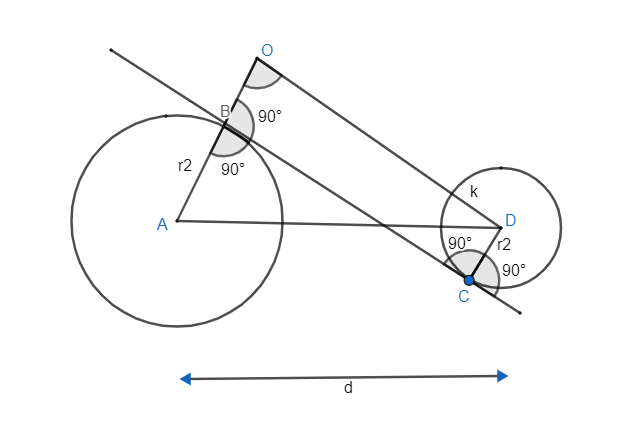
Now, as we know radius and tangent are perpendicular to each other at the point of tangency. And we also know there is only one perpendicular direction for any line that is direction of perpendicular to any line will be unique.
As,
Now, proceed the radius AB (which is parallel to CD) to the length of CD that is
As
Hence, ODCB will be rectangle with equal opposite sides and all the angles of it will be
So,
Now, we know
In
As, OBCD is a rectangle, so
Hence, we can write above equation as
Now, coming to the question, we are given that radii of two circles are 8 cm and 3 cm and distance between centres is 13 cm. So, we get length of common transverse tangent from the equation (2) as
Hence, the length of the transverse common tangent is
Note: Don’t get confused with the direct and transverse tangent. Direct tangent will look like as
So, be clear with both.
Extending AB to O such that the length BO is equal to the radius of the other circle is the key point of the question.
One may think that we cannot solve the problem without supporting some points in coordinate form. So, try not to include any coordinates. It is possible to solve the problem without imposing any coordinates.
(i) Radius is perpendicular to tangent at the point of tangency
(ii) Radius of both the circles at the tangency point will be parallel to each other.
Length of common transverse tangent is given as
Complete step-by-step answer:
Here, we have two circles with radii 8 cm and 3 cm, and we need to determine the length of the transverse common tangent, if the distance between centres of both the circles is 13 cm.
So, length of transverse tangent can be calculated

Now, as we know radius and tangent are perpendicular to each other at the point of tangency. And we also know there is only one perpendicular direction for any line that is direction of perpendicular to any line will be unique.
As,
Now, proceed the radius AB (which is parallel to CD) to the length of CD that is
As
Hence, ODCB will be rectangle with equal opposite sides and all the angles of it will be
So,
Now, we know
In
As, OBCD is a rectangle, so
Hence, we can write above equation as
Now, coming to the question, we are given that radii of two circles are 8 cm and 3 cm and distance between centres is 13 cm. So, we get length of common transverse tangent from the equation (2) as
Hence, the length of the transverse common tangent is
Note: Don’t get confused with the direct and transverse tangent. Direct tangent will look like as

So, be clear with both.
Extending AB to O such that the length BO is equal to the radius of the other circle is the key point of the question.
One may think that we cannot solve the problem without supporting some points in coordinate form. So, try not to include any coordinates. It is possible to solve the problem without imposing any coordinates.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




