
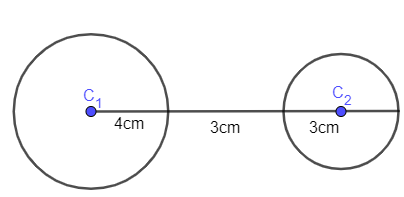
Find the length of the transverse common tangent.
(a)
(b) 7
(c)
(d)

Answer
526.8k+ views
Hint: Transverse common tangent (TCT) meets on the line passing through the centre. Find the distance between the centre of the circle and mark it as ‘
Complete step-by-step answer:
A common tangent is a line that is tangent to two circles. Now a transverse common tangent meets on the line passing through the centre and divides it internally in the ratio of radii as shown in figure.
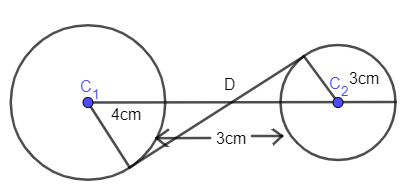
So, let us mark D as the point where the common tangent meets the line passing the centre of the circle.
We have been given the two circles as
The length of a transverse common tangent to two circles is given by the formula,
Length of a transverse common tangent
Here, we know ‘d’ as the distance between centres. Hence, from the figure
d=10cm
Now let us apply all the values in (i)
Length of a transverse common tangent
Hence, we got the length of the transverse common tangent as
Note: In the circle
Complete step-by-step answer:
A common tangent is a line that is tangent to two circles. Now a transverse common tangent meets on the line passing through the centre and divides it internally in the ratio of radii as shown in figure.

So, let us mark D as the point where the common tangent meets the line passing the centre of the circle.
We have been given the two circles as
The length of a transverse common tangent to two circles is given by the formula,
Length of a transverse common tangent
Here, we know ‘d’ as the distance between centres. Hence, from the figure
d=10cm
Now let us apply all the values in (i)
Length of a transverse common tangent
Hence, we got the length of the transverse common tangent as
Note: In the circle
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




