
How do you find the length, width, and height of a rectangular prism if the volume is
Answer
476.1k+ views
Hint: A polynomial is factored completely when it is expressed as a product of one or more polynomials that cannot be factored further. To factor a polynomial completely, we need to identify the greatest common monomial factor. Not all polynomials can be factored in. We know that the volume of a rectangular prism is
Complete step by step answer:
As per the given question, we have to find the dimensions of the rectangular prism using factoring methods by factoring the given volume expression. Here, we have the given volume expression
Let a rectangular prism with the dimension’s length ‘l’, width ‘b’ and height ‘h’ as shown in the figure below:
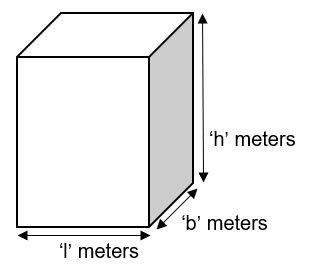
Then, the volume of the prism is given by
Here, we can observe that ‘h’ is common on both sides of the equation. Thus, we can eliminate ‘h’ to get
In the quadratic equation
Taking
As we know that length is greater than width, then we get
Note:
In order to solve these types of questions, we need to have enough knowledge of factoring methods of polynomials. If polynomials can’t be factored then we can use quadratic formula
Complete step by step answer:
As per the given question, we have to find the dimensions of the rectangular prism using factoring methods by factoring the given volume expression. Here, we have the given volume expression
Let a rectangular prism with the dimension’s length ‘l’, width ‘b’ and height ‘h’ as shown in the figure below:

Then, the volume of the prism is given by
Here, we can observe that ‘h’ is common on both sides of the equation. Thus, we can eliminate ‘h’ to get
In the quadratic equation
Taking
As we know that length is greater than width, then we get
Note:
In order to solve these types of questions, we need to have enough knowledge of factoring methods of polynomials. If polynomials can’t be factored then we can use quadratic formula
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Earth rotates from West to east ATrue BFalse class 6 social science CBSE

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Dr BR Ambedkars fathers name was Ramaji Sakpal and class 10 social science CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE




