
How do I find the limit as
Answer
468.6k+ views
Hint: In order to find the solution to this question, we will first convert the term in limits form and then find the limits. We are using limits because infinity is not a number as
Complete step by step answer:
From the question, we can see that we have been asked to find the value of
So, to start with the solution, we will first convert the given statement into a mathematical expression. Therefore, we can convert into limits since infinity is not a number.
Therefore, we get:
As we can see above, x approaches to negative infinity, therefore the answer is undefined because
If the scenario is:
x approaches infinity that is positive infinity, then:
That is the limit of the natural logarithm of
We can understand with the help of the following graph:
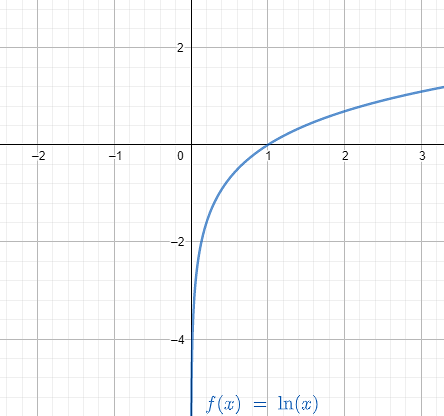
As when
In this case, the natural logarithm of minus infinity is undefined for real numbers, since the natural logarithm function is undefined for negative numbers:
Therefore, we get:
We can understand with the help of the following graph:
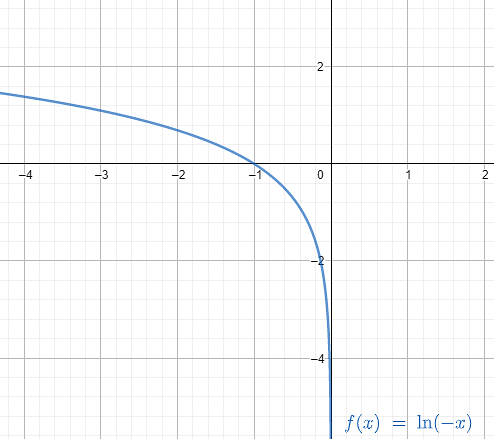
Therefore, we can conclude at this point:
And
Note:
In a hurry, we might end up reading the question wrong and then getting the wrong answer. So, we have to be very careful about that. Also, we can solve this question from the graph of ln x, but sometimes we get confused about the graph, so we have used the conventional method to find the answer.
Complete step by step answer:
From the question, we can see that we have been asked to find the value of
So, to start with the solution, we will first convert the given statement into a mathematical expression. Therefore, we can convert into limits since infinity is not a number.
Therefore, we get:
As we can see above, x approaches to negative infinity, therefore the answer is undefined because
If the scenario is:
x approaches infinity that is positive infinity, then:
That is the limit of the natural logarithm of
We can understand with the help of the following graph:

As when
In this case, the natural logarithm of minus infinity is undefined for real numbers, since the natural logarithm function is undefined for negative numbers:
Therefore, we get:
We can understand with the help of the following graph:

Therefore, we can conclude at this point:
And
Note:
In a hurry, we might end up reading the question wrong and then getting the wrong answer. So, we have to be very careful about that. Also, we can solve this question from the graph of ln x, but sometimes we get confused about the graph, so we have used the conventional method to find the answer.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




