
Find the local maxima and minima values of
Answer
386.7k+ views
Hint: This question involves the use of differentiation. To find the function's local minimum or maximum value, we need to find the critical points using differentiation and double differentiate the final equation to confirm whether the point will give maxima or minima.
For minima, the conditions are:
For maxima, the conditions are:
Complete answer:
So, let us consider the function
Differentiating both sides with respect to x, we get,
Using quotient rule of differentiation
Now, using the product rule of differentiation
Now, we know the power rule of differentiation
Opening brackets and simplifying the expression, we get,
Adding up like terms, we get,
Simplifying the equation by opening the brackets, we get,
Adding up like terms, we get,
To find the critical points, we have to equate the derivative of the function to zero. Cross multiplying the terms, we get,
Splitting the middle term of the quadratic equation to get the roots, we get,
Taking common terms outside the bracket,
So, the points of local extremum are:
Now, to check whether critical points return the local minima or the local maxima we need to again differentiate the function and put
So, differentiating both sides of equation
Using quotient rule, we get,
Using the power rule of differentiation
Now, substituting the value of x as
Simplifying the calculations,
Since the second derivative is negative,
Hence, local maxima value is
Simplifying the calculations,
So, the local maxima value of
Now, we substitute x as
Simplifying the calculations, we get,
So, the second derivative of the function at
So, to obtain the local minima of the function, we substitute
So,
Hence, the local maxima value of
Note:
The local maxima can also be less than the local minima, as in this case if the function is not continuous over the real number line. This would become more clear with the graphical representation of the function
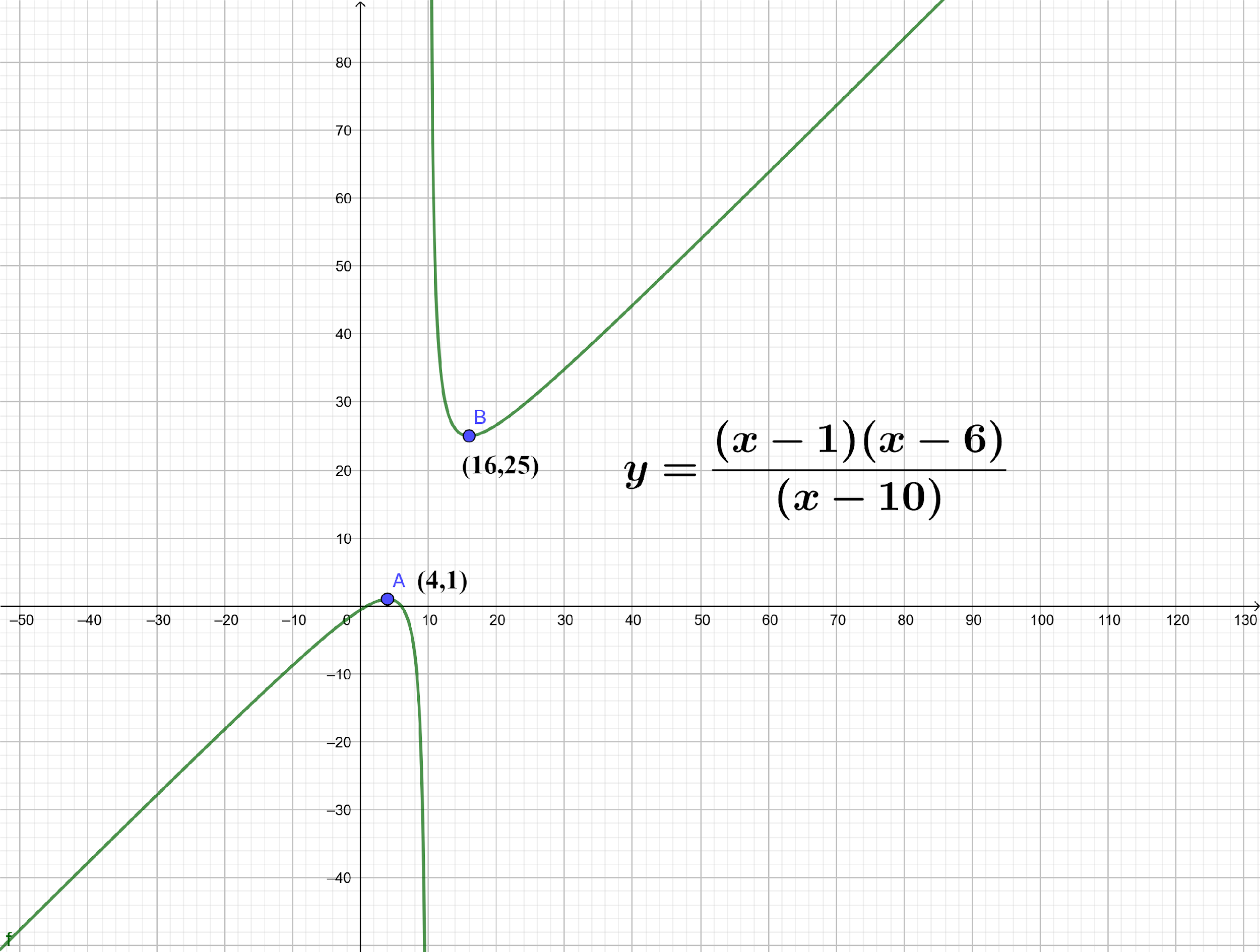
The quotient rule, product rule, chain rule and power rule of differentiation must be remembered before solving such advanced applications of derivatives problems.
For minima, the conditions are:
For maxima, the conditions are:
Complete answer:
So, let us consider the function
Differentiating both sides with respect to x, we get,
Using quotient rule of differentiation
Now, using the product rule of differentiation
Now, we know the power rule of differentiation
Opening brackets and simplifying the expression, we get,
Adding up like terms, we get,
Simplifying the equation by opening the brackets, we get,
Adding up like terms, we get,
To find the critical points, we have to equate the derivative of the function to zero. Cross multiplying the terms, we get,
Splitting the middle term of the quadratic equation to get the roots, we get,
Taking common terms outside the bracket,
So, the points of local extremum are:
Now, to check whether critical points return the local minima or the local maxima we need to again differentiate the function and put
So, differentiating both sides of equation
Using quotient rule, we get,
Using the power rule of differentiation
Now, substituting the value of x as
Simplifying the calculations,
Since the second derivative is negative,
Hence, local maxima value is
Simplifying the calculations,
So, the local maxima value of
Now, we substitute x as
Simplifying the calculations, we get,
So, the second derivative of the function at
So, to obtain the local minima of the function, we substitute
So,
Hence, the local maxima value of
Note:
The local maxima can also be less than the local minima, as in this case if the function is not continuous over the real number line. This would become more clear with the graphical representation of the function

The quotient rule, product rule, chain rule and power rule of differentiation must be remembered before solving such advanced applications of derivatives problems.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




