
Find the magnetic field at point P due to a straight line segment
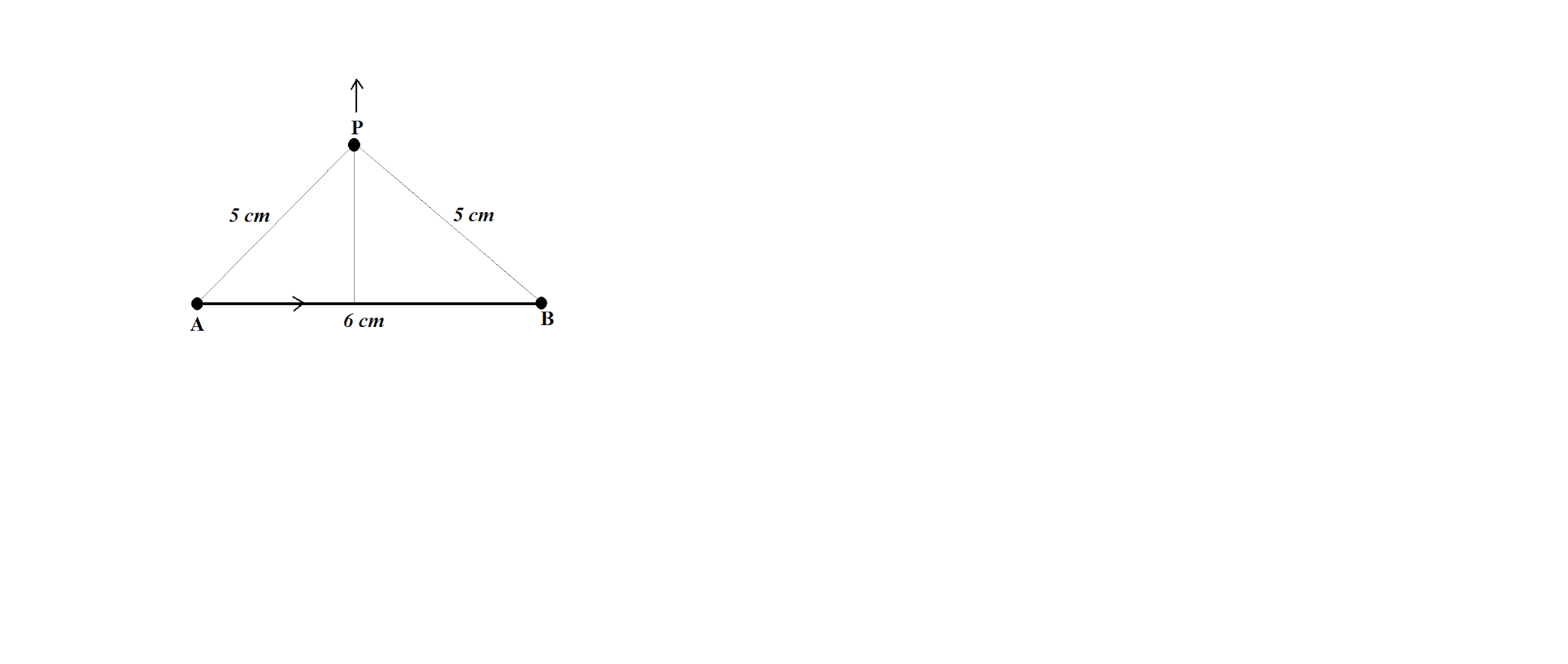

Answer
507.6k+ views
Hint: To find the value of magnetic field at point P, we will use the formula of magnetic field due to a straight current carrying wire segment at a point present on the equatorial axis of the segment.
Formula used:
Complete step by step answer:
Magnetic field is a vector field, or pseudo vector field, that describes the magnetic influence or impact of electric charges in relative motion with each other and effect of magnetized materials. A charge moving parallel to a current of other charges experiences a force perpendicular to its own velocity. We can say that the magnetic field is the area around a magnet in which there is presence of magnetic force. Magnetic field is a type of field that passes through space and which makes a magnetic force move electric charges and magnetic dipoles.
Suppose MN is a straight conductor carrying a current
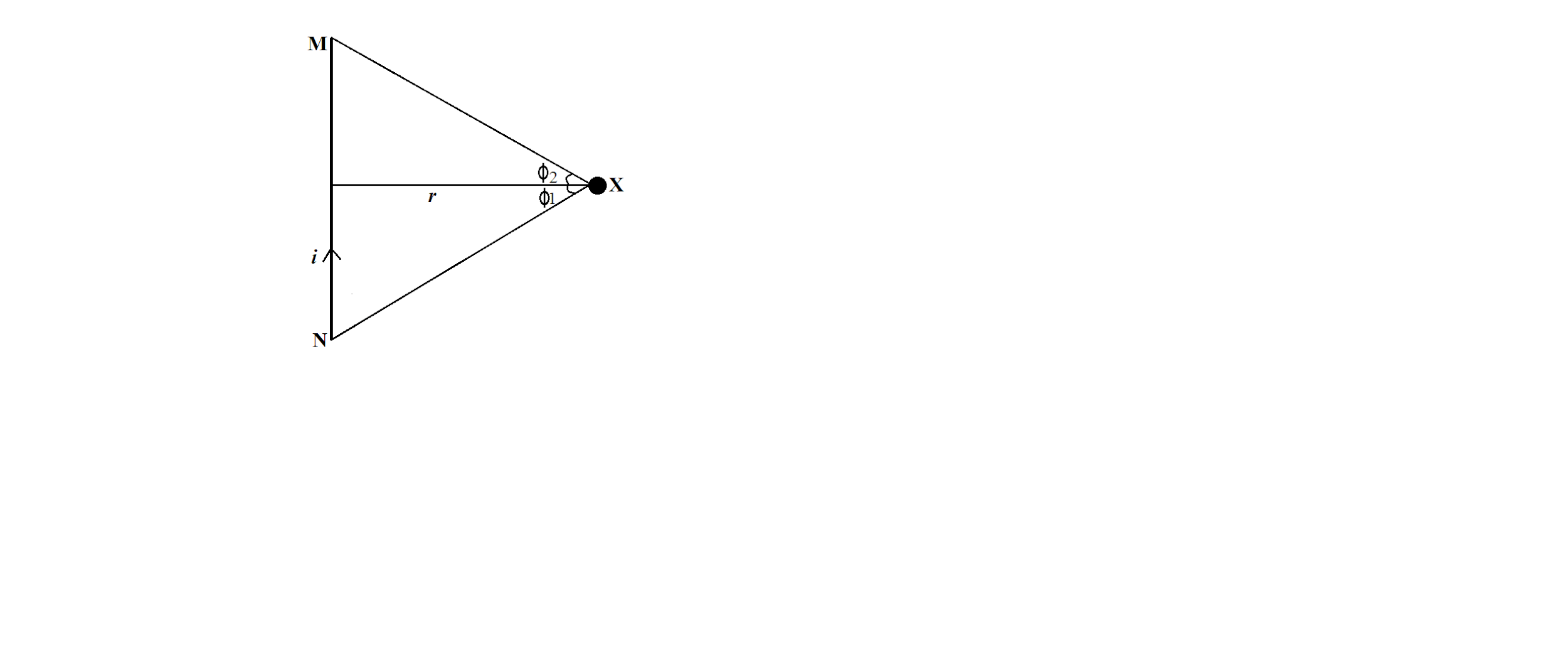
According to Biot-Savart law, magnetic field at point X is,
Angle between
Now,
And,
We get,
Therefore,
Also,
Total magnetic field at point X due to entire conductor is,
Magnetic field due to a finite current carrying wire is given by,
We are given a wire segment
Magnetic field due to a finite current carrying wire is given by,
Distance
Current
Angle
Putting all the values, we get,
Value of Magnetic field at point P due to straight line segment
Hence, the correct option is D.
Note: Students should note that the value of
Formula used:
Complete step by step answer:
Magnetic field is a vector field, or pseudo vector field, that describes the magnetic influence or impact of electric charges in relative motion with each other and effect of magnetized materials. A charge moving parallel to a current of other charges experiences a force perpendicular to its own velocity. We can say that the magnetic field is the area around a magnet in which there is presence of magnetic force. Magnetic field is a type of field that passes through space and which makes a magnetic force move electric charges and magnetic dipoles.
Suppose MN is a straight conductor carrying a current

According to Biot-Savart law, magnetic field at point X is,
Angle between
Now,
And,
We get,
Therefore,
Also,
Total magnetic field at point X due to entire conductor is,
Magnetic field due to a finite current carrying wire is given by,
We are given a wire segment
Magnetic field due to a finite current carrying wire is given by,
Distance
Current
Angle
Putting all the values, we get,
Value of Magnetic field at point P due to straight line segment
Hence, the correct option is D.
Note: Students should note that the value of
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




