Question

Find the magnitude in radians and degrees of the interior angle of
(1) A regular pentagon
(2) A regular heptagon
(3) A regular octagon
(4) A regular dodecagon and,
(5) A regular polygon of 17 sides.

Answer 1

Hint: We will apply the formula for interior angle which is given by $\dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}$ where n is called the number of sides of a regular polygon. We can substitute the value of n for corresponding polygons and then find the interior angles. We will get it in degrees, after which we can use the conversion given by ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$ to find the angle in radians.

Complete step-by-step answer:
A polygon is any shape made by a number of sides. The interior angle is an angle which is made by the sides inside that polygon. Numerically, the formula for interior angle is given by $\dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}$ where n is called the number of sides of a regular polygon. And since, the polygon is regular therefore all its interior angles will be the same.
A regular pentagon is simply a polygon with n = 5 sides having the same interior angles. Now, we will apply the formula for interior angle is given by $\dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}$ where n is called the number of sides of a regular polygon. Since, we have n = 5 thus, we get
$\begin{align}
  & \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{180}^{\text{o}}}\left( 5-2 \right)}{5} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{36}^{\text{o}}}\left( 3 \right)}{1} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}={{\left( 36\times 3 \right)}^{\text{o}}} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}={{108}^{\text{o}}} \\
\end{align}$
Clearly, every interior angle of a regular pentagon is 108 degrees.
Now, we will convert it into radians by using the formula given by ${{\left( \pi \right)}^{c}}={{180}^{\circ }}$ or ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$. As ${{108}^{\text{o}}}=108\times {{\left( 1 \right)}^{\text{o}}}$. Therefore, by using the formula ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$ we have,
$\begin{align}
  & {{108}^{\text{o}}}=108\times {{\left( 1 \right)}^{\text{o}}} \\
 & \Rightarrow {{108}^{\text{o}}}=108\times {{\left( \dfrac{\pi }{180} \right)}^{c}} \\
 & \Rightarrow {{108}^{\text{o}}}={{\left( 108\times \dfrac{\pi }{180} \right)}^{c}} \\
 & \Rightarrow {{108}^{\text{o}}}={{\left( 3\times \dfrac{\pi }{5} \right)}^{c}} \\
 & \Rightarrow {{108}^{\text{o}}}={{\left( \dfrac{3\pi }{5} \right)}^{c}} \\
\end{align}$
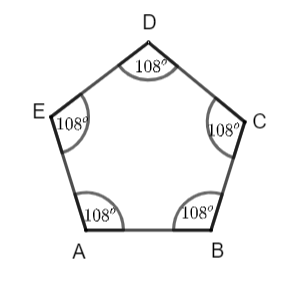
And clearly, every interior angle of a regular pentagon is ${{\left( \dfrac{3\pi }{5} \right)}^{c}}$. The diagram of pentagon is shown below.

Now, we will consider a heptagon. Now, we will apply the formula for interior angle is given by $\dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}$ where n is called the number of sides of a regular polygon. Since, we have n = 7 thus, we get
$\begin{align}
  & \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{180}^{\text{o}}}\left( 7-2 \right)}{7} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{180}^{\text{o}}}\left( 5 \right)}{7} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}={{\left( \dfrac{180\times 5}{7} \right)}^{\text{o}}} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}={{\left( \dfrac{900}{7} \right)}^{\text{o}}} \\
\end{align}$
Clearly, every interior angle of a regular heptagon is ${{\left( \dfrac{900}{7} \right)}^{\text{o}}}$ or, ${{\left( 128.5 \right)}^{\text{o}}}$.
Now, we will convert it into radians by using the formula given by ${{\left( \pi \right)}^{c}}={{180}^{\circ }}$ or ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$. As ${{\left( \dfrac{900}{7} \right)}^{\text{o}}}=\dfrac{900}{7}\times {{\left( 1 \right)}^{\text{o}}}$. Therefore, by using the formula ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$ we have,
$\begin{align}
  & {{\left( \dfrac{900}{7} \right)}^{\text{o}}}=\dfrac{900}{7}\times {{\left( 1 \right)}^{\text{o}}} \\
 & \Rightarrow {{\left( \dfrac{900}{7} \right)}^{\text{o}}}=\dfrac{900}{7}\times {{\left( \dfrac{\pi }{180} \right)}^{c}} \\
 & \Rightarrow {{\left( \dfrac{900}{7} \right)}^{\text{o}}}={{\left( \dfrac{900}{7}\times \dfrac{\pi }{180} \right)}^{c}} \\
 & \Rightarrow {{\left( \dfrac{900}{7} \right)}^{\text{o}}}={{\left( \dfrac{5}{7}\times \dfrac{\pi }{1} \right)}^{c}} \\
 & \Rightarrow {{\left( \dfrac{900}{7} \right)}^{\text{o}}}={{\left( \dfrac{5\pi }{7} \right)}^{c}} \\
\end{align}$
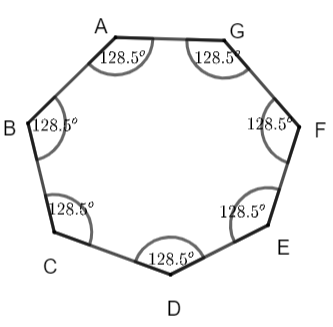
And clearly, every interior angle of a heptagon is ${{\left( \dfrac{5\pi }{7} \right)}^{c}}$. The diagram of heptagon is shown below.

Now, we will consider an octagon. Now, we will apply the formula for interior angle is given by $\dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}$ where n is called the number of sides of a regular polygon. Since, we have n = 8 thus, we get
$\begin{align}
  & \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{180}^{\text{o}}}\left( 8-2 \right)}{8} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{180}^{\text{o}}}\left( 6 \right)}{8} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{45}^{\text{o}}}\left( 3 \right)}{1} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}={{\left( 45\times 3 \right)}^{\text{o}}} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}={{135}^{\text{o}}} \\
\end{align}$
Clearly, every interior angle of an octagon is ${{135}^{\text{o}}}$.
Now, we will convert it into radians by using the formula given by ${{\left( \pi \right)}^{c}}={{180}^{\circ }}$ or ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$. As ${{135}^{\text{o}}}=135\times {{\left( 1 \right)}^{\text{o}}}$. Therefore, by using the formula ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$ we have,
$\begin{align}
  & {{135}^{\text{o}}}=135\times {{\left( 1 \right)}^{\text{o}}} \\
 & \Rightarrow {{135}^{\text{o}}}=135\times {{\left( \dfrac{\pi }{180} \right)}^{c}} \\
 & \Rightarrow {{135}^{\text{o}}}={{\left( 135\times \dfrac{\pi }{180} \right)}^{c}} \\
 & \Rightarrow {{135}^{\text{o}}}={{\left( 3\times \dfrac{\pi }{4} \right)}^{c}} \\
 & \Rightarrow {{135}^{\text{o}}}={{\left( \dfrac{3\pi }{4} \right)}^{c}} \\
\end{align}$
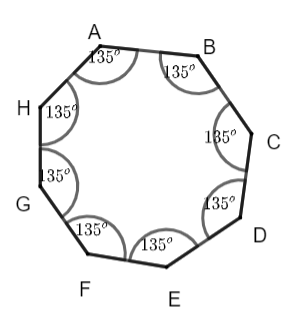
And clearly, every interior angle of an octagon is ${{\left( \dfrac{3\pi }{4} \right)}^{c}}$. The diagram of octagon is shown below.

A duo decagon is a polygon with 12 sides. It is also known as dodecagon. Now, we will consider a duo decagon. Now, we will apply the formula for interior angle is given by $\dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}$ where n is called the number of sides of a regular polygon. Since, we have n = 12 thus, we get
$\begin{align}
  & \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{180}^{\text{o}}}\left( 12-2 \right)}{12} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{180}^{\text{o}}}\left( 10 \right)}{12} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}={{150}^{\text{o}}} \\
\end{align}$
Clearly, every interior angle of a duo decagon is ${{150}^{\text{o}}}$.
Now, we will convert it into radians by using the formula given by ${{\left( \pi \right)}^{c}}={{180}^{\circ }}$ or ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$. As ${{150}^{\text{o}}}=150\times {{\left( 1 \right)}^{\text{o}}}$. Therefore, by using the formula ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$ we have,
$\begin{align}
  & {{150}^{\text{o}}}=150\times {{\left( 1 \right)}^{\text{o}}} \\
 & \Rightarrow {{150}^{\text{o}}}=150\times {{\left( \dfrac{\pi }{180} \right)}^{c}} \\
 & \Rightarrow {{150}^{\text{o}}}={{\left( 150\times \dfrac{\pi }{180} \right)}^{c}} \\
 & \Rightarrow {{150}^{\text{o}}}={{\left( 5\times \dfrac{\pi }{6} \right)}^{c}} \\
 & \Rightarrow {{150}^{\text{o}}}={{\left( \dfrac{5\pi }{6} \right)}^{c}} \\
\end{align}$
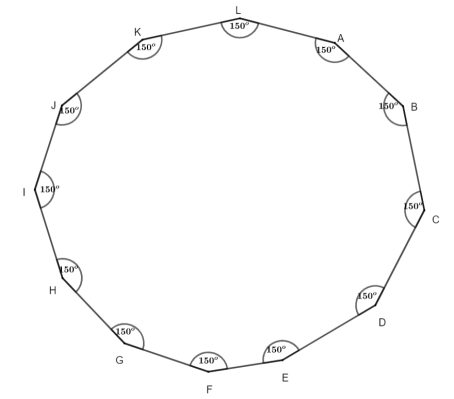
And clearly, every interior angle of a duo decagon is ${{\left( \dfrac{5\pi }{6} \right)}^{c}}$. The diagram of duo decagon is shown below.

Now, we will consider a regular polygon of 17 sides. Now, we will apply the formula for interior angle is given by $\dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}$ where n is called the number of sides of a regular polygon. Since, we have n = 17 thus, we get
$\begin{align}
  & \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{180}^{\text{o}}}\left( 17-2 \right)}{17} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}=\dfrac{{{180}^{\text{o}}}\left( 15 \right)}{17} \\
 & \Rightarrow \dfrac{{{180}^{\text{o}}}\left( n-2 \right)}{n}={{\left( \dfrac{2700}{17} \right)}^{\text{o}}} \\
\end{align}$
Clearly, every interior angle of a regular polygon of 17 sides is ${{\left( \dfrac{2700}{17} \right)}^{\text{o}}}$.
Now, we will convert it into radians by using the formula given by ${{\left( \pi \right)}^{c}}={{180}^{\circ }}$ or ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$. As ${{\left( \dfrac{2700}{17} \right)}^{\text{o}}}=\dfrac{2700}{17}\times {{\left( 1 \right)}^{\text{o}}}$. Therefore, by using the formula ${{\left( 1 \right)}^{\text{o}}}={{\left( \dfrac{\pi }{180} \right)}^{c}}$ we have,
$\begin{align}
  & {{\left( \dfrac{2700}{17} \right)}^{\text{o}}}=\dfrac{2700}{17}\times {{\left( 1 \right)}^{\text{o}}} \\
 & \Rightarrow {{\left( \dfrac{2700}{17} \right)}^{\text{o}}}=\dfrac{2700}{17}\times {{\left( \dfrac{\pi }{180} \right)}^{c}} \\
 & \Rightarrow {{\left( \dfrac{2700}{17} \right)}^{\text{o}}}={{\left( \dfrac{2700}{17}\times \dfrac{\pi }{180} \right)}^{c}} \\
 & \Rightarrow {{\left( \dfrac{2700}{17} \right)}^{\text{o}}}={{\left( 15\times \dfrac{\pi }{17} \right)}^{c}} \\
 & \Rightarrow {{\left( \dfrac{2700}{17} \right)}^{\text{o}}}={{\left( \dfrac{15\pi }{17} \right)}^{c}} \\
\end{align}$
And clearly, every interior angle of a regular polygon of 17 sides is ${{\left( \dfrac{15\pi }{17} \right)}^{c}}$. The diagram of a regular polygon of 17 sides can be drawn with the help of above diagrams with 17 sides.

Note: The names of the polygons according to the number of sides are discussed in note. The three sides of polygon is called triangle, the 4 sides polygon is called quadrilateral (it can be square or rectangle or rhombus), polygon with 5 sides is pentagon, 6 sides polygon is hexagon, and then heptagon with 7 sides, octagon with 8 sides. It is important to know this otherwise it will be difficult to solve this question. Also, we must read the question carefully, there is a chance that we might miss that part of finding angles in degrees as well as radians.