
How do you find the midpoint and length of a line segment in a coordinate plane?
Answer
442.8k+ views
1 likes
Hint: In the given question, we are required to find the midpoint and length of the line segment in the coordinate plane. In order to determine the length and midpoint of a line segment, we must know the coordinates of the end points of the segment. There are various methods of finding the midpoint and distance of a line segment.
Complete step by step solution:
coordinates of the both end points of the line segment.
Let the line segment be AB and the coordinates of the end points be
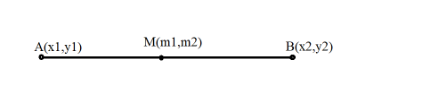
Now, we can find the midpoint of the line segment AB using the midpoint formula and coordinates of the endpoints of the line segment AB given to us.
So, according to the midpoint formula, coordinates of the midpoint of a line segment are
So, to find the coordinates of the midpoint of line segment AB, we calculate the average of the x-coordinates and the average of the y-coordinates of the endpoints.
So, the coordinates of the midpoint are:
Now, we have to find the length of the line segment AB.
So, let the length of the line segment be d.
Then, we can find the distance of a line segment given the endpoints of the segment using the distance formula as:
So, the length of the line segment is
Note: If the coordinates of the endpoints of the given line segment are in three dimensions, then we can apply the same distance formula and midpoint formula with slight variations.
The midpoint formula for three dimensions is
Complete step by step solution:
coordinates of the both end points of the line segment.
Let the line segment be AB and the coordinates of the end points be

Now, we can find the midpoint of the line segment AB using the midpoint formula and coordinates of the endpoints of the line segment AB given to us.
So, according to the midpoint formula, coordinates of the midpoint of a line segment are
So, to find the coordinates of the midpoint of line segment AB, we calculate the average of the x-coordinates and the average of the y-coordinates of the endpoints.
So, the coordinates of the midpoint are:
Now, we have to find the length of the line segment AB.
So, let the length of the line segment be d.
Then, we can find the distance of a line segment given the endpoints of the segment using the distance formula as:
So, the length of the line segment is
Note: If the coordinates of the endpoints of the given line segment are in three dimensions, then we can apply the same distance formula and midpoint formula with slight variations.
The midpoint formula for three dimensions is
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




