
Find the net elongation of the composite rod (Assume A = cross section of each rod).
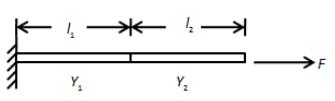
A.
B.
C.
D.
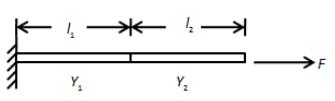
Answer
483.3k+ views
Hint:Recall the expression for the Young’s modulus and express the elongation produced in the both rods separately. The change in the length of the composite rod is equal to the sum of elongation of the respective lengths of the rod. Substitute the elongations of the both lengths and determine the net elongation of the composite rod.
Formula used:
Here, F is the applied force, l is the original length of the rod, A is the cross-sectional area of the rod and
Complete step by step answer:
We assume there is a small elongation
We have the expression for the Young’s modulus is,
Here, F is the applied force, l is the original length of the rod, A is the cross-sectional area of the rod and
We can express the small elongation produced in the rod of length
We can also express the small elongation produced in the rod of length
These small elongations in the lengths
Using equation (1) and (2) in the above equation, we get,
So, the correct answer is option B.
Note:The composite rod is formed by joining the two metal rods of different materials. The Young’s modulus depends on the property of the material and therefore, the Young’s modulus is different for the two rods. The elongation produced in the rod depends on the Young’s modulus of the rod and therefore, the elongation is different for the two rods.
Formula used:
Here, F is the applied force, l is the original length of the rod, A is the cross-sectional area of the rod and
Complete step by step answer:
We assume there is a small elongation
We have the expression for the Young’s modulus is,
Here, F is the applied force, l is the original length of the rod, A is the cross-sectional area of the rod and
We can express the small elongation produced in the rod of length
We can also express the small elongation produced in the rod of length
These small elongations in the lengths
Using equation (1) and (2) in the above equation, we get,
So, the correct answer is option B.
Note:The composite rod is formed by joining the two metal rods of different materials. The Young’s modulus depends on the property of the material and therefore, the Young’s modulus is different for the two rods. The elongation produced in the rod depends on the Young’s modulus of the rod and therefore, the elongation is different for the two rods.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




