
Find the number of divisors of 8064.
Answer
536.7k+ views
Hint: Apply prime factorisation to find the prime factors of the given number and then apply formula to find the number of divisors of the given number.
The given number is 8064.
First we find all the prime factors of the given number.
Prime factors are numbers which are divisible by itself, that is, it has ‘one’ and itself as the factors.
Now let’s find all the prime factors of 8064 using prime factorisation method
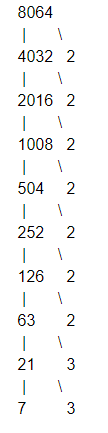
So, 8064 can be written as,
By grouping, we get
Now to find the number of divisors we will use the formula,
Where ‘n’ is the number and ‘p, q, r’ are the powers of prime factors of the numbers or they are the exponents of prime factorisation.
Such that a number can be represented as,
Where ‘a, b, c’ are prime factors of ‘n’
Now applying this formula in our problem, we get
Comparing equation (1) and (2), we get
P=7, q=2, r=1
So the number of divisors will be
Solving we get
So the number of divisors of 8064 is 48.
Note: Student forgets to add one in the divisor formula i.e.,
Will be mistakenly written as,
The answer we get in both cases does not match and the student will end up in the wrong answer if he uses formula (B) instead of (A).
The given number is 8064.
First we find all the prime factors of the given number.
Prime factors are numbers which are divisible by itself, that is, it has ‘one’ and itself as the factors.
Now let’s find all the prime factors of 8064 using prime factorisation method
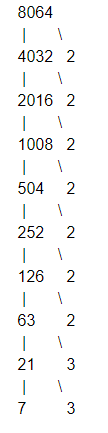
So, 8064 can be written as,
By grouping, we get
Now to find the number of divisors we will use the formula,
Where ‘n’ is the number and ‘p, q, r’ are the powers of prime factors of the numbers or they are the exponents of prime factorisation.
Such that a number can be represented as,
Where ‘a, b, c’ are prime factors of ‘n’
Now applying this formula in our problem, we get
Comparing equation (1) and (2), we get
P=7, q=2, r=1
So the number of divisors will be
Solving we get
So the number of divisors of 8064 is 48.
Note: Student forgets to add one in the divisor formula i.e.,
Will be mistakenly written as,
The answer we get in both cases does not match and the student will end up in the wrong answer if he uses formula (B) instead of (A).
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




