Find the orthocenter of the triangle with the equation of its sides are $x+y=1$, $2x+3y=0,4x-y+4=0$.
Answer
Verified471.9k+ views
Hint: First of all draw a triangle ABC and find the intersections of any two pair of equations given above and find the coordinates of A and B then write the equation of an altitude drawn from point A and also write the equation of an altitude drawn from point B and then the intersection of the two altitudes from point A and B is the orthocenter of triangle ABC.
Complete step-by-step solution:
In the below diagram, we have drawn a triangle ABC with equations of its sides.
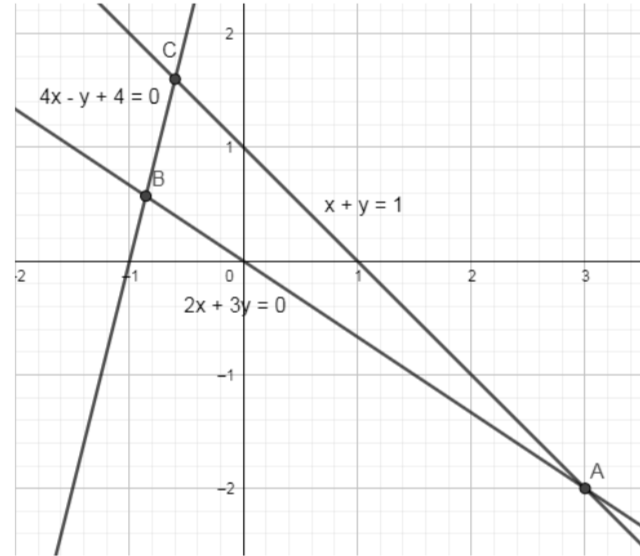
We are going to find the coordinates of point A by the intersection of the following equations:
$\begin{align}
& x+y=1..........Eq.(1) \\
& 2x+3y=0..........Eq.(2) \\
\end{align}$
Multiplying first equation by 3 and then subtracting second equation from first equation we get,
$\begin{align}
& \left( x+y=1 \right)\times 3 \\
& -\left( 2x+3y=0 \right) \\
\end{align}$
\[\begin{align}
& 3x+3y=3 \\
& \dfrac{-2x-3y=0}{x=3} \\
\end{align}\]
Substituting $x=3$ in eq. (1) we get,
$\begin{align}
& x+y=1 \\
& \Rightarrow 3+y=1 \\
& \Rightarrow y=-2 \\
\end{align}$
Hence, we have got the coordinates of point A (3, -2).
Now, we are going to find the coordinates of point B by the intersections of equations:
$\begin{align}
& 2x+3y=0......Eq.(2) \\
& 4x-y+4=0.......Eq.(3) \\
\end{align}$
Multiplying eq. (3) by 3 and then adding this eq. (3) into eq. (2) we get,
$\begin{align}
& \left( 4x-y+4=0 \right)\times 3 \\
& 2x+3y=0 \\
\end{align}$
$\begin{align}
& 12x-3y+12=0 \\
& +\dfrac{\left( 2x+3y=0 \right)}{14x+0+12=0} \\
\end{align}$
Rearranging the above equation we get,
$\begin{align}
& 14x=-12 \\
& \Rightarrow x=-\dfrac{12}{14}=-\dfrac{6}{7} \\
\end{align}$
Substituting the above value of x in eq. (2) we get,
$\begin{align}
& 2x+3y=0 \\
& \Rightarrow 2\left( -\dfrac{6}{7} \right)+3y=0 \\
& \Rightarrow -\dfrac{12}{7}+3y=0 \\
& \Rightarrow 3y=\dfrac{12}{7} \\
\end{align}$
Dividing 3 on both the sides of the above equation we get,
$y=\dfrac{4}{7}$
Hence, we have got the coordinates of point B as $\left( -\dfrac{6}{7},\dfrac{4}{7} \right)$.
Now, we will find the equation of altitudes passing through A and B.
In the below figure, we have shown altitudes passing through A and B and the intersection of altitudes is point F.
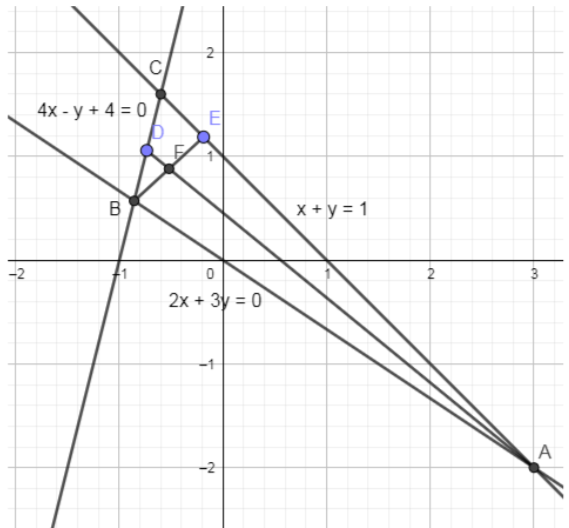
As altitude is making right angle on the opposite side from the vertex it has drawn so equation of altitude passing through vertex A is calculated as follows:
As altitude from point A and side BC is perpendicular to each other so slope of altitude we can find as follows:
The equation of side BC is equal to $4x-y+4=0$. The slope of this line is calculated by the following formula:
$m=-\dfrac{\text{coefficient of x}}{\text{coefficient of y}}$
In the equation of side BC, coefficient of x is 4 and coefficient of y is – 1 so substituting these values in the above equation we get,
$\begin{align}
& m=-\dfrac{4}{\left( -1 \right)} \\
& \Rightarrow m=4 \\
\end{align}$
We know that when two lines with slopes ${{m}_{1}}\And {{m}_{2}}$ are perpendicular then the relationship between their slopes is equal to:
${{m}_{1}}{{m}_{2}}=-1$
We know the slope of side BC as 4 so substituting this slope value in the above equation we get the slope of altitude passing through vertex A.
$\begin{align}
& \left( 4 \right){{m}_{2}}=-1 \\
& \Rightarrow {{m}_{2}}=-\dfrac{1}{4} \\
\end{align}$
Now, we have got the slope of the equation of altitude passing through A and we know that general form of equation of a line is:
$y=mx+c$…… Eq. (4)
In the above formula, m is the slope and c is the y intercept of the line so substituting the value of slope as $-\dfrac{1}{4}$ in the above equation we get,
$y=\left( -\dfrac{1}{4} \right)x+c$………Eq. (5)
Now, this line is passing through vertex A (3, -2) so substituting this point in the above equation by writing x as 3 and y as -2 in the above equation we get,
$\begin{align}
& -2=\left( -\dfrac{1}{4} \right)3+c \\
& \Rightarrow -2=-\dfrac{3}{4}+c \\
\end{align}$
Adding $\dfrac{3}{4}$ on both the sides of the above equation we get,
$\begin{align}
& -2+\dfrac{3}{4}=c \\
& \Rightarrow \dfrac{-8+3}{4}=c \\
& \Rightarrow \dfrac{-5}{4}=c \\
\end{align}$
Substituting the above value of c in eq. (5) we get,
$y=\left( -\dfrac{1}{4} \right)x-\dfrac{5}{4}$
$\begin{align}
& \Rightarrow 4y=-x-5 \\
& \Rightarrow x+4y+5=0 \\
\end{align}$
Hence, we have got the equation of an altitude passing through vertex A as $x+4y+5=0$.
Similarly, we can find the equation of an altitude passing through vertex B $\left( -\dfrac{6}{7},\dfrac{4}{7} \right)$.
The altitude through point B rests on side CA. Slope of the side CA $x+y=1$ is equal to -1 so using the relationship between slopes of two perpendicular lines we can find the slope of altitude passing through vertex B is:
$\begin{align}
& \left( -1 \right){{m}_{2}}=-1 \\
& \Rightarrow {{m}_{2}}=1 \\
\end{align}$
The general form of an equation of a line is:
$y=mx+c$
Substituting the value of m as 1 we get,
$y=x+c$ …….. Eq. (6)
Substituting the point B $\left( -\dfrac{6}{7},\dfrac{4}{7} \right)$ in the above equation we get,
$\begin{align}
& \dfrac{4}{7}=-\dfrac{6}{7}+c \\
& \Rightarrow c=\dfrac{10}{7} \\
\end{align}$
Substituting the above value of c in eq. (6) we get,
$y=x+\dfrac{10}{7}$
We have got the equation of an altitude passing through point B is equal to:
$y=x+\dfrac{10}{7}$………Eq. (7)
We have already shown the equation of an altitude passing through point A is $x+4y+5=0$.
Now, the intersection of these two altitudes is an orthocenter so we are going to find the intersection of these altitudes by substituting the value of y from eq. (7) in $x+4y+5=0$ we get,
$\begin{align}
& x+4\left( x+\dfrac{10}{7} \right)+5=0 \\
& \Rightarrow x+4x+\dfrac{40}{7}+5=0 \\
& \Rightarrow 5x+\dfrac{40+35}{7}=0 \\
& \Rightarrow 5x=-\dfrac{75}{7} \\
& \Rightarrow x=-\dfrac{15}{7} \\
\end{align}$
Substituting the above value of x in eq. (7) we get,
$\begin{align}
& y=-\dfrac{15}{7}+\dfrac{10}{7} \\
& \Rightarrow y=-\dfrac{5}{7} \\
\end{align}$
Hence, the orthocenter of triangle ABC is $\left( -\dfrac{15}{7},-\dfrac{5}{7} \right)$.
Note: We can verify that the orthocenter that we are getting is correct or not by substituting this orthocenter in the equation of any altitude that we have solved above.
Let us take equation of an altitude from vertex A which is:
$x+4y+5=0$
Substituting the orthocenter as $\left( -\dfrac{15}{7},-\dfrac{5}{7} \right)$ in the above equation we get,
$\begin{align}
& -\dfrac{15}{7}+4\left( \dfrac{-5}{7} \right)+5=0 \\
& \Rightarrow \dfrac{-15-20}{7}+5=0 \\
& \Rightarrow -\dfrac{35}{7}+5=0 \\
& \Rightarrow -5+5=0 \\
& \Rightarrow 0=0 \\
\end{align}$
As you can see that L.H.S = R.H.S so the orthocenter that we have solved above is correct.
Complete step-by-step solution:
In the below diagram, we have drawn a triangle ABC with equations of its sides.
We are going to find the coordinates of point A by the intersection of the following equations:
$\begin{align}
& x+y=1..........Eq.(1) \\
& 2x+3y=0..........Eq.(2) \\
\end{align}$
Multiplying first equation by 3 and then subtracting second equation from first equation we get,
$\begin{align}
& \left( x+y=1 \right)\times 3 \\
& -\left( 2x+3y=0 \right) \\
\end{align}$
\[\begin{align}
& 3x+3y=3 \\
& \dfrac{-2x-3y=0}{x=3} \\
\end{align}\]
Substituting $x=3$ in eq. (1) we get,
$\begin{align}
& x+y=1 \\
& \Rightarrow 3+y=1 \\
& \Rightarrow y=-2 \\
\end{align}$
Hence, we have got the coordinates of point A (3, -2).
Now, we are going to find the coordinates of point B by the intersections of equations:
$\begin{align}
& 2x+3y=0......Eq.(2) \\
& 4x-y+4=0.......Eq.(3) \\
\end{align}$
Multiplying eq. (3) by 3 and then adding this eq. (3) into eq. (2) we get,
$\begin{align}
& \left( 4x-y+4=0 \right)\times 3 \\
& 2x+3y=0 \\
\end{align}$
$\begin{align}
& 12x-3y+12=0 \\
& +\dfrac{\left( 2x+3y=0 \right)}{14x+0+12=0} \\
\end{align}$
Rearranging the above equation we get,
$\begin{align}
& 14x=-12 \\
& \Rightarrow x=-\dfrac{12}{14}=-\dfrac{6}{7} \\
\end{align}$
Substituting the above value of x in eq. (2) we get,
$\begin{align}
& 2x+3y=0 \\
& \Rightarrow 2\left( -\dfrac{6}{7} \right)+3y=0 \\
& \Rightarrow -\dfrac{12}{7}+3y=0 \\
& \Rightarrow 3y=\dfrac{12}{7} \\
\end{align}$
Dividing 3 on both the sides of the above equation we get,
$y=\dfrac{4}{7}$
Hence, we have got the coordinates of point B as $\left( -\dfrac{6}{7},\dfrac{4}{7} \right)$.
Now, we will find the equation of altitudes passing through A and B.
In the below figure, we have shown altitudes passing through A and B and the intersection of altitudes is point F.
As altitude is making right angle on the opposite side from the vertex it has drawn so equation of altitude passing through vertex A is calculated as follows:
As altitude from point A and side BC is perpendicular to each other so slope of altitude we can find as follows:
The equation of side BC is equal to $4x-y+4=0$. The slope of this line is calculated by the following formula:
$m=-\dfrac{\text{coefficient of x}}{\text{coefficient of y}}$
In the equation of side BC, coefficient of x is 4 and coefficient of y is – 1 so substituting these values in the above equation we get,
$\begin{align}
& m=-\dfrac{4}{\left( -1 \right)} \\
& \Rightarrow m=4 \\
\end{align}$
We know that when two lines with slopes ${{m}_{1}}\And {{m}_{2}}$ are perpendicular then the relationship between their slopes is equal to:
${{m}_{1}}{{m}_{2}}=-1$
We know the slope of side BC as 4 so substituting this slope value in the above equation we get the slope of altitude passing through vertex A.
$\begin{align}
& \left( 4 \right){{m}_{2}}=-1 \\
& \Rightarrow {{m}_{2}}=-\dfrac{1}{4} \\
\end{align}$
Now, we have got the slope of the equation of altitude passing through A and we know that general form of equation of a line is:
$y=mx+c$…… Eq. (4)
In the above formula, m is the slope and c is the y intercept of the line so substituting the value of slope as $-\dfrac{1}{4}$ in the above equation we get,
$y=\left( -\dfrac{1}{4} \right)x+c$………Eq. (5)
Now, this line is passing through vertex A (3, -2) so substituting this point in the above equation by writing x as 3 and y as -2 in the above equation we get,
$\begin{align}
& -2=\left( -\dfrac{1}{4} \right)3+c \\
& \Rightarrow -2=-\dfrac{3}{4}+c \\
\end{align}$
Adding $\dfrac{3}{4}$ on both the sides of the above equation we get,
$\begin{align}
& -2+\dfrac{3}{4}=c \\
& \Rightarrow \dfrac{-8+3}{4}=c \\
& \Rightarrow \dfrac{-5}{4}=c \\
\end{align}$
Substituting the above value of c in eq. (5) we get,
$y=\left( -\dfrac{1}{4} \right)x-\dfrac{5}{4}$
$\begin{align}
& \Rightarrow 4y=-x-5 \\
& \Rightarrow x+4y+5=0 \\
\end{align}$
Hence, we have got the equation of an altitude passing through vertex A as $x+4y+5=0$.
Similarly, we can find the equation of an altitude passing through vertex B $\left( -\dfrac{6}{7},\dfrac{4}{7} \right)$.
The altitude through point B rests on side CA. Slope of the side CA $x+y=1$ is equal to -1 so using the relationship between slopes of two perpendicular lines we can find the slope of altitude passing through vertex B is:
$\begin{align}
& \left( -1 \right){{m}_{2}}=-1 \\
& \Rightarrow {{m}_{2}}=1 \\
\end{align}$
The general form of an equation of a line is:
$y=mx+c$
Substituting the value of m as 1 we get,
$y=x+c$ …….. Eq. (6)
Substituting the point B $\left( -\dfrac{6}{7},\dfrac{4}{7} \right)$ in the above equation we get,
$\begin{align}
& \dfrac{4}{7}=-\dfrac{6}{7}+c \\
& \Rightarrow c=\dfrac{10}{7} \\
\end{align}$
Substituting the above value of c in eq. (6) we get,
$y=x+\dfrac{10}{7}$
We have got the equation of an altitude passing through point B is equal to:
$y=x+\dfrac{10}{7}$………Eq. (7)
We have already shown the equation of an altitude passing through point A is $x+4y+5=0$.
Now, the intersection of these two altitudes is an orthocenter so we are going to find the intersection of these altitudes by substituting the value of y from eq. (7) in $x+4y+5=0$ we get,
$\begin{align}
& x+4\left( x+\dfrac{10}{7} \right)+5=0 \\
& \Rightarrow x+4x+\dfrac{40}{7}+5=0 \\
& \Rightarrow 5x+\dfrac{40+35}{7}=0 \\
& \Rightarrow 5x=-\dfrac{75}{7} \\
& \Rightarrow x=-\dfrac{15}{7} \\
\end{align}$
Substituting the above value of x in eq. (7) we get,
$\begin{align}
& y=-\dfrac{15}{7}+\dfrac{10}{7} \\
& \Rightarrow y=-\dfrac{5}{7} \\
\end{align}$
Hence, the orthocenter of triangle ABC is $\left( -\dfrac{15}{7},-\dfrac{5}{7} \right)$.
Note: We can verify that the orthocenter that we are getting is correct or not by substituting this orthocenter in the equation of any altitude that we have solved above.
Let us take equation of an altitude from vertex A which is:
$x+4y+5=0$
Substituting the orthocenter as $\left( -\dfrac{15}{7},-\dfrac{5}{7} \right)$ in the above equation we get,
$\begin{align}
& -\dfrac{15}{7}+4\left( \dfrac{-5}{7} \right)+5=0 \\
& \Rightarrow \dfrac{-15-20}{7}+5=0 \\
& \Rightarrow -\dfrac{35}{7}+5=0 \\
& \Rightarrow -5+5=0 \\
& \Rightarrow 0=0 \\
\end{align}$
As you can see that L.H.S = R.H.S so the orthocenter that we have solved above is correct.
Recently Updated Pages
Class 10 Question and Answer - Your Ultimate Solutions Guide
Master Class 10 Science: Engaging Questions & Answers for Success
Master Class 10 Maths: Engaging Questions & Answers for Success
Master Class 10 General Knowledge: Engaging Questions & Answers for Success
Master Class 10 Social Science: Engaging Questions & Answers for Success
Master Class 10 English: Engaging Questions & Answers for Success
Trending doubts
What is Commercial Farming ? What are its types ? Explain them with Examples
List out three methods of soil conservation
Complete the following word chain of verbs Write eat class 10 english CBSE
Compare and contrast a weekly market and a shopping class 10 social science CBSE
Imagine that you have the opportunity to interview class 10 english CBSE
On the outline map of India mark the following appropriately class 10 social science. CBSE