
Find the perimeter of a triangle with sides
Answer
490.8k+ views
Hint: The perimeter of a plane surface is the length of its boundaries. Thus, the perimeter of a triangle is the sum of all of its sides. Add the given sides of the triangle to determine its perimeter.
Complete step-by-step answer:
According to the given question, the sides of the triangle are
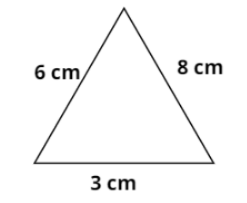
We know that the perimeter of a plane surface is the length of its boundaries.
But since a triangle consists of three sides, its perimeter will be the sum of all of its sides. Therefore using this we will get the perimeter as:
Thus the perimeter of the triangle is 17 cm.
Additional Information:
If the area of the triangle is asked instead, then we can use the formula:
If all the sides of the triangle are known then Heron’s formula can also be used to determine its area. This formula is:
If the triangle is a right angled triangle instead, then its area can be calculated by the formula:
Note: For three lines to form a triangle, the sum of the lengths of any two of them must be greater than the length of the third one. In the above question, the lengths of the sides of the triangle are
Therefore the condition is valid for a triangle.
Complete step-by-step answer:
According to the given question, the sides of the triangle are

We know that the perimeter of a plane surface is the length of its boundaries.
But since a triangle consists of three sides, its perimeter will be the sum of all of its sides. Therefore using this we will get the perimeter as:
Thus the perimeter of the triangle is 17 cm.
Additional Information:
If the area of the triangle is asked instead, then we can use the formula:
If all the sides of the triangle are known then Heron’s formula can also be used to determine its area. This formula is:
If the triangle is a right angled triangle instead, then its area can be calculated by the formula:
Note: For three lines to form a triangle, the sum of the lengths of any two of them must be greater than the length of the third one. In the above question, the lengths of the sides of the triangle are
Therefore the condition is valid for a triangle.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

How many ten lakhs are in one crore-class-8-maths-CBSE





