
Find the perimeter of
(i) A regular pentagon of side 8 cm.
(ii) A regular octagon of side 4.5 cm.
(iii) A regular decagon of side 3.6 cm.
Answer
443.5k+ views
2 likes
Hint: To solve this question, we should know what a regular polygon is. A regular polygon is a polygon that is equilateral and equiangular. So, all the sides of the polygon will be the same. We will use the given side to find the sum of the sides of the given pentagon, octagon and decagon respectively to find their perimeters.
Complete step-by-step answer:
We have been asked to find the perimeter of a regular pentagon, octagon and decagon. We have been given the measure of 1 side of each of them. Before we start solving the question, we should know that a regular polygon is one that is equilateral and equiangular. So, it means that it will have the same measure for all its sides. And we know that the perimeter is calculated as the sum of all the sides. So, we will use this concept to find the perimeter of these.
(i) A regular pentagon of side 8 cm.
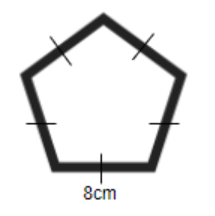
We know that a regular pentagon will have 5 sides that are of the same length. So, let us take the length of the side of the pentagon, which is given as
(ii) A regular octagon of side 4.5 cm.
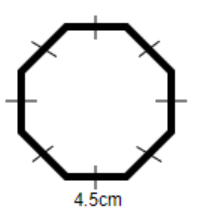
Now, we know that a regular octagon will have 8 equal sides. So, let us assume the length of the side of the octagon as
(iii) A regular decagon of side 3.6 cm.
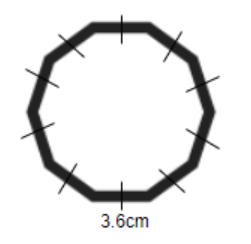
We know that a regular decagon has 10 equal sides. So, we will take the length of the side of the regular decagon as
Therefore, we get the perimeters of the regular pentagon as 40 cm, octagon as 36 cm and decagon as 36 cm.
Note: We have considered the formula for the perimeters as 5a, 8b and 10c for the pentagon, octagon and decagon respectively as they were regular polygons and the sides were equal. If the perimeters were not regular polygons, then we cannot use this formula for finding the perimeter.
Complete step-by-step answer:
We have been asked to find the perimeter of a regular pentagon, octagon and decagon. We have been given the measure of 1 side of each of them. Before we start solving the question, we should know that a regular polygon is one that is equilateral and equiangular. So, it means that it will have the same measure for all its sides. And we know that the perimeter is calculated as the sum of all the sides. So, we will use this concept to find the perimeter of these.
(i) A regular pentagon of side 8 cm.

We know that a regular pentagon will have 5 sides that are of the same length. So, let us take the length of the side of the pentagon, which is given as
(ii) A regular octagon of side 4.5 cm.

Now, we know that a regular octagon will have 8 equal sides. So, let us assume the length of the side of the octagon as
(iii) A regular decagon of side 3.6 cm.

We know that a regular decagon has 10 equal sides. So, we will take the length of the side of the regular decagon as
Therefore, we get the perimeters of the regular pentagon as 40 cm, octagon as 36 cm and decagon as 36 cm.
Note: We have considered the formula for the perimeters as 5a, 8b and 10c for the pentagon, octagon and decagon respectively as they were regular polygons and the sides were equal. If the perimeters were not regular polygons, then we cannot use this formula for finding the perimeter.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
What is the full form of AD a After death b Anno domini class 6 social science CBSE

How many millions make a billion class 6 maths CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

Four bells toll together at 900am They toll after 7811 class 6 maths CBSE

Name the countries which are larger than India class 6 social science CBSE

How many lightyears away is the sun from the earth class 6 social science CBSE





