Question

Find the value of cos (135°).

Answer 1

Hint:There are 4 quadrants in the trigonometric plane. Figure out in which quadrant does the given angle lie on. ALL, SINE, TAN, COS are positive in the order of quadrant 1,2,3 and 4 anticlockwise. The rest trigonometric functions are negative.

Complete step by step solution:
135° clearly lies in the 2 nd quadrant. All trigonometric functions except “sine” are negative in the 2 nd quadrant.

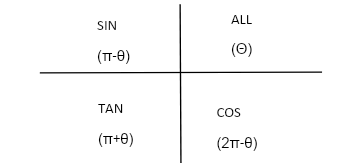
Let, 135° = Θ.
As we can see that whenever the required angle (Θ) is in 2 nd quadrant, we can write the angle as (π-
θ).

Therefore, cos (135°) = - cos (π-135°)

We know that π= 180°
∴ cos (135°) = - cos (π-135°) = - cos (180°−135°)
= - cos (45°)
= $ - \dfrac{1}{{\sqrt 2 }} $

Alternate method: Instead of the above steps, you might also write cos (135°) as the sum of two standard angles,
cos (135°) = cos (45°+90°)

Using the formula, cos (A+B) = cos A. cos B – sin A. sin B
= cos 45°. cos 90° - sin 45°. sin 90°
= $ \dfrac{1}{{\sqrt 2 }} $ . 0 - $ \dfrac{1}{{\sqrt 2 }} $ . 1 = - $ \dfrac{1}{{\sqrt 2 }} $

Note: Whenever there are trigonometric calculations to be done, the quadrants must be kept in mind. The signs play a major role in determining the correct answer. One might use the first approach to write in exams, and the second approach when such short questions are given to be answered in a very short time.