
How to find the value of
Answer
418.5k+ views
Hint: To find the value of
Complete step-by-step solution:
The word Trigonometry is derived from the Greek word trigon and metron. Here trigon means figures with three angles and metron means measurement. That is the meaning of the word Trigonometry is a measurement of triangles. In the modern age Trigonometry has broad based meaning. In simple words we define it as that branch of mathematics which deals with the measurement of angles and the problems allied with angles.
Currently trigonometry is used in many areas such as the science of seismology, designing electric circuits, predicting the heights of tides in the ocean, analyzing the musical tone and in many other areas.
We know that the angle between
A series of real valued functions, defined as the ratio of the sides of a triangle is called a Trigonometric Function.
Trigonometric functions are also known as the circular functions.The basic trigonometric functions are sine, cosine, tangent, cotangent, secant and cosecant. The angles of sine, cosine, and tangent are the primary classification of functions of trigonometry. And the three functions which are cotangent, secant and cosecant can be derived from the primary functions.
Now according to the question to find the value of
We know that
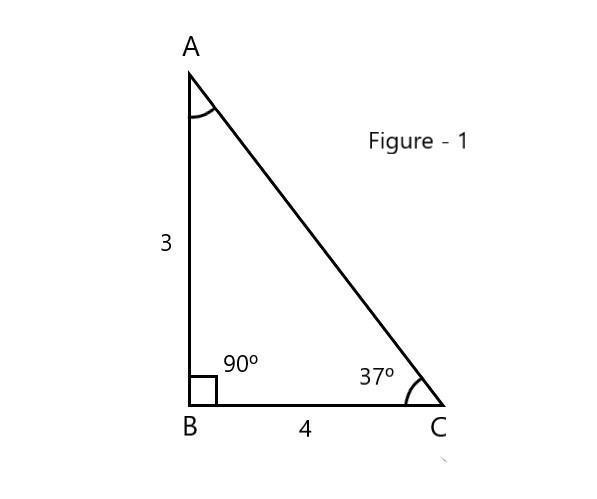
Now from
By angle sum property:
Let
Now by Pythagoras theorem:
According to figure
We know that
Hence,
Now to find the value of
Apply the formula
Where
We know that
Put this value in above equation we get ,
Hence
Note: We must keep one thing in mind that
Complete step-by-step solution:
The word Trigonometry is derived from the Greek word trigon and metron. Here trigon means figures with three angles and metron means measurement. That is the meaning of the word Trigonometry is a measurement of triangles. In the modern age Trigonometry has broad based meaning. In simple words we define it as that branch of mathematics which deals with the measurement of angles and the problems allied with angles.
Currently trigonometry is used in many areas such as the science of seismology, designing electric circuits, predicting the heights of tides in the ocean, analyzing the musical tone and in many other areas.
We know that the angle between
A series of real valued functions, defined as the ratio of the sides of a triangle is called a Trigonometric Function.
Trigonometric functions are also known as the circular functions.The basic trigonometric functions are sine, cosine, tangent, cotangent, secant and cosecant. The angles of sine, cosine, and tangent are the primary classification of functions of trigonometry. And the three functions which are cotangent, secant and cosecant can be derived from the primary functions.
Now according to the question to find the value of
We know that

Now from
By angle sum property:
Let
Now by Pythagoras theorem:
According to figure
We know that
Hence,
Now to find the value of
Apply the formula
Where
We know that
Put this value in above equation we get ,
Hence
Note: We must keep one thing in mind that
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




