
Find the value of
Answer
517.2k+ views
2 likes
Hint: To determine the square root of a number we can either use the long division method or the prime factorisation method. As here in the question we are asked to find the root of 7 to six decimal places, long division method would be a wise choice.
Complete step-by-step answer:
For numbers like
Now double the quotient you got in the first step and make it the tenth place of a number, now find a unit place to the number such that the number into its unit place is equal to or closest to the number you obtained as the remainder of the previous steps. Also, make the unit digit of the number, the unit digit of the quotient as well. Repeat the steps till you get the remainder zero. However, if you introduce a decimal point to the quotient, unlike normal division instead of one zero, pair of zeroes are introduced at the end of the remainder of the previous step.
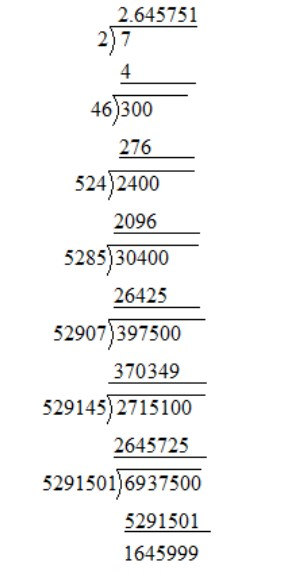
Therefore, the root of 7 is 2.645751, correct up to six decimal places.
Note: While calculating square roots and cube roots, prime factorization is the easiest method. But it takes time and difficult to use for the questions where the square roots are not integers. Hence, other methods should also be learnt, so that they can be used while solving problems in cases where time plays an important role.
Complete step-by-step answer:
For numbers like
Now double the quotient you got in the first step and make it the tenth place of a number, now find a unit place to the number such that the number into its unit place is equal to or closest to the number you obtained as the remainder of the previous steps. Also, make the unit digit of the number, the unit digit of the quotient as well. Repeat the steps till you get the remainder zero. However, if you introduce a decimal point to the quotient, unlike normal division instead of one zero, pair of zeroes are introduced at the end of the remainder of the previous step.
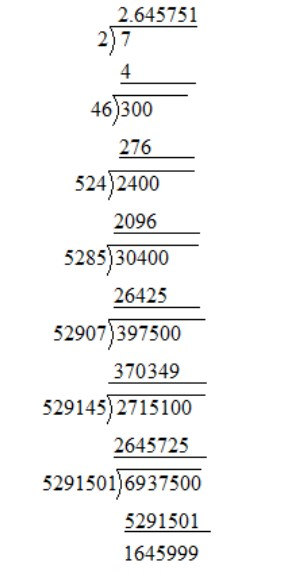
Therefore, the root of 7 is 2.645751, correct up to six decimal places.
Note: While calculating square roots and cube roots, prime factorization is the easiest method. But it takes time and difficult to use for the questions where the square roots are not integers. Hence, other methods should also be learnt, so that they can be used while solving problems in cases where time plays an important role.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




