
Answer
479.1k+ views
Hint: In order to find the value of the polynomial p(x) at x= a, we calculate the value of each of the terms of f(x) at x= a and hence find the value of p(x) at x= a.
Complete step-by-step answer:
[i] x =0
Substituting x = 0 in the expression of p(x), we get
$5\left( 0 \right)-4{{\left( 0 \right)}^{2}}+3=0-0+3=3$
Hence the value of p(x) at x= 0 is 3
[ii] x = -1
Substituting x = 1 in the expression of p(x), we get
$5\left( -1 \right)-4{{\left( -1 \right)}^{2}}+3=-5-4+3=-6$
Hence the value of p(x) at x = -1 is -6
[iii] x = 2
Substituting x = 2 in the expression of p(x), we get
$5\left( 2 \right)-4{{\left( 2 \right)}^{2}}+3=10-16+3=-3$
Hence the value of p(x) at x= 2 is -3
Note: Alternative method: Synthetic division: Best method.
In this method, we start by writing coefficients of the polynomial in order from the highest degree to the constant term. If in between some degree terms are missing we set their coefficient as 0.
Hence $p\left( x \right)=5x-4{{x}^{2}}+3=-4{{x}^{2}}+5x+3$ will be written as
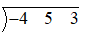
Now the point which has to be substituted(say x= 0) is written as follows
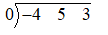
0 is placed below the first term
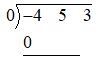
Now the terms under the same column are added. The sum is then multiplied with the root, and the product is written under the coefficient of the next term.
Hence, we have
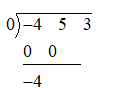
Continuing in this way we have the following
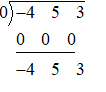
Since the last sum is 3, we have $p\left( 0 \right)=3$. This method also tells you what the quotient will be when p(x) is divided by x-0. Here it will be $-4\left( x \right)+5=-4x+5$
Similarly creating tables for x = -1 and x=2 , we get

Since the last sum is -6, we have $p\left( -1 \right)=-6$
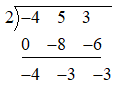
Since the last sum is -3, we have $p\left( 2 \right)=-3$
Complete step-by-step answer:
[i] x =0
Substituting x = 0 in the expression of p(x), we get
$5\left( 0 \right)-4{{\left( 0 \right)}^{2}}+3=0-0+3=3$
Hence the value of p(x) at x= 0 is 3
[ii] x = -1
Substituting x = 1 in the expression of p(x), we get
$5\left( -1 \right)-4{{\left( -1 \right)}^{2}}+3=-5-4+3=-6$
Hence the value of p(x) at x = -1 is -6
[iii] x = 2
Substituting x = 2 in the expression of p(x), we get
$5\left( 2 \right)-4{{\left( 2 \right)}^{2}}+3=10-16+3=-3$
Hence the value of p(x) at x= 2 is -3
Note: Alternative method: Synthetic division: Best method.
In this method, we start by writing coefficients of the polynomial in order from the highest degree to the constant term. If in between some degree terms are missing we set their coefficient as 0.
Hence $p\left( x \right)=5x-4{{x}^{2}}+3=-4{{x}^{2}}+5x+3$ will be written as
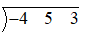
Now the point which has to be substituted(say x= 0) is written as follows
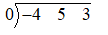
0 is placed below the first term
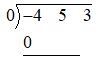
Now the terms under the same column are added. The sum is then multiplied with the root, and the product is written under the coefficient of the next term.
Hence, we have
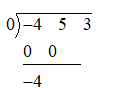
Continuing in this way we have the following
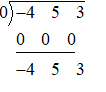
Since the last sum is 3, we have $p\left( 0 \right)=3$. This method also tells you what the quotient will be when p(x) is divided by x-0. Here it will be $-4\left( x \right)+5=-4x+5$
Similarly creating tables for x = -1 and x=2 , we get

Since the last sum is -6, we have $p\left( -1 \right)=-6$
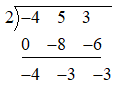
Since the last sum is -3, we have $p\left( 2 \right)=-3$
Recently Updated Pages
10 Examples of Evaporation in Daily Life with Explanations

10 Examples of Diffusion in Everyday Life

1 g of dry green algae absorb 47 times 10 3 moles of class 11 chemistry CBSE

If x be real then the maximum value of 5 + 4x 4x2 will class 10 maths JEE_Main

If the coordinates of the points A B and C be 443 23 class 10 maths JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Who was the leader of the Bolshevik Party A Leon Trotsky class 9 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Which is the largest saltwater lake in India A Chilika class 8 social science CBSE

Ghatikas during the period of Satavahanas were aHospitals class 6 social science CBSE




