
Find the value of the unknown interior angle x in the following figure.
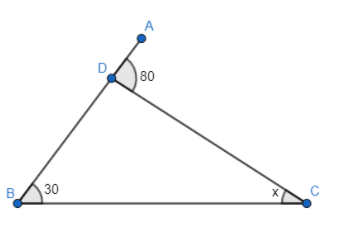

Answer
498.9k+ views
Hint: Use the property that the exterior angle of a triangle is equal to the sum of the opposite interior angles of a triangle. So, for the above figure,
Complete step-by-step answer:
Let us start the solution to the above question by drawing the diagram given in the question.
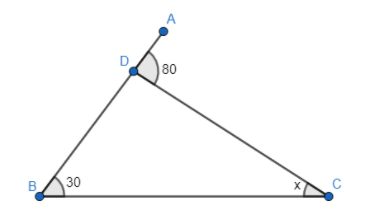
We know that the exterior angle of a triangle is equal to the sum of the opposite interior angles of a triangle. In the above figure,
If we take 30 to the other side of the equation, the sign changes. So, we get
Therefore, we can conclude that the measure of x is equal to
Note:Generally, it is seen that whenever a student is asked about the exterior angle of the triangle, they misinterpret it as the corresponding interior angle subtracted from
Complete step-by-step answer:
Let us start the solution to the above question by drawing the diagram given in the question.

We know that the exterior angle of a triangle is equal to the sum of the opposite interior angles of a triangle. In the above figure,
If we take 30 to the other side of the equation, the sign changes. So, we get
Therefore, we can conclude that the measure of x is equal to
Note:Generally, it is seen that whenever a student is asked about the exterior angle of the triangle, they misinterpret it as the corresponding interior angle subtracted from
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

Write five sentences about Earth class 8 biology CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE

Advantages and disadvantages of science

In a school there are two sections of class X section class 8 maths CBSE




