Question

Find the value of trigonometric functions.

Answer 1

Hint: Such question is to be done on the basic quadrant rule of trigonometry.
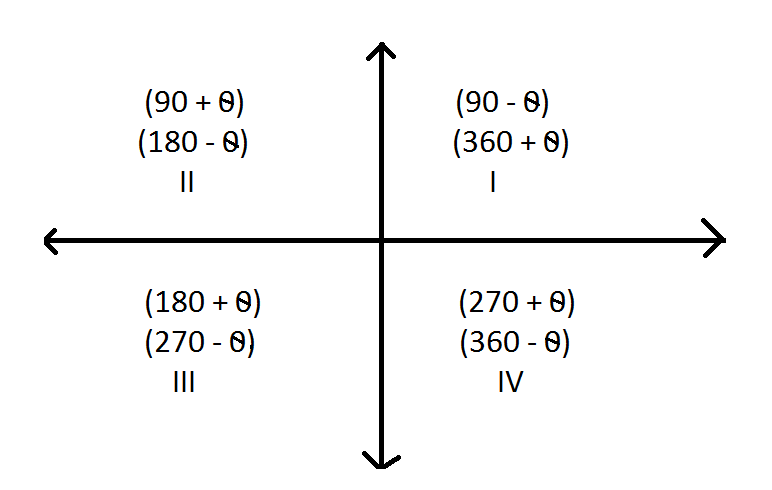
In the first quadrant the values of \[\sin ,\cos ,\tan \]and \[\cot \] are positive. In the second quadrant \[\sin \,and\,\cos ec\] are positive. In the third quadrant \[\tan \] and the\[\cot \]and positive and in the fourth quadrant \[\cos \] and \[\sec \] are positive.
Trigonometric ratio’s do change at odd multiples of \[{90^0},{270^0},{450^0}\]etc
Example \[\operatorname{Sin} (90 - \theta ) = \operatorname{Cos} \theta \]
At odd multiples of \[{90^0}\operatorname{Sin} \] change to \[\cos \] and \[\cos \] to \[\sin ,\tan \] changes to \[\cot \] and \[\cot \]changes to \[\tan ,\] \[\cos es\] changes to \[\sec \] and \[\sec \] changes to \[\cos es\]
Trigonometric ratios do not change at even multiples of \[{90^0}\,\] i.e. \[180,360\]etc.
They remain same
In first quadrant\[\theta \] lies between \[O < \theta < 90\]
In second quadrant\[\theta \] lies between \[90 < O < 180\]
In third quadrant\[\theta \] lies between \[180 < \theta < 270\]
In fourth quadrant\[\theta \] lies between \[360 < \theta < 270\]

Therefore,

Complete step by step answer:
\[\sin {765^0}.........(1)\]
We can write \[765 = 720 + 45\]
\[ \Rightarrow 765 = 2 \times 360 + 45...........eqn(2)\]
Using the equation (2) in (1)
We have, \[(\sin ){765^0} = \operatorname{Sin} \left[ { = 2(360) + 45} \right]\]
We know \[360 + \theta \] lies in the first quadrant and in the first quadrant all trigonometric ratios are positive.
Also \[360\] is an even multiple of \[90\]
Hence \[\operatorname{Sin} (360 + \theta ) = \operatorname{Sin} \theta \]
i.e. \[Sin\left[ {2(360) + 45)} \right] = \operatorname{Sin} 45\]

Note: The quadrant rule can be used to find the trigonometric ratio of any angle. In the first round it covers \[{360^0}\]. Second \[{720^0}\] and so on.
All the trigonometric ratio is converted into standard angles, whose values are known through the trigonometric table.