
Find the value of

Answer
400.8k+ views
Hint:To solve this question we have to use the concept which tells that if we start from one place and return to that same place on a circular boundary then the covered angle is
Complete step by step answer:
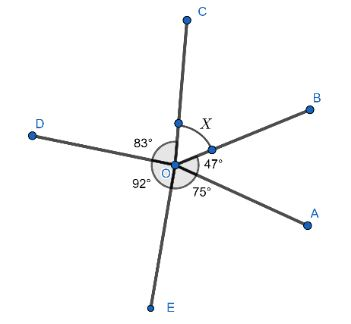
Given, a fig is given in which angles are given.
To find the unknown angle which is given in the angle.
To solve this equation sum of all the angles are equal to
On putting all the values from the given things.
On further adding all the angles.
Taking
On further solving
Hence, the value of the unknown angle is
Note:To solve this type of question you must know the angles made by a single line incomplete revolution and the angle between two string lines and two perpendicular lines. And if all angles are given in terms of a single variable then try to find the value of that single variable. and apply that to all the relations of the angles and find the values of all known angles.
Complete step by step answer:
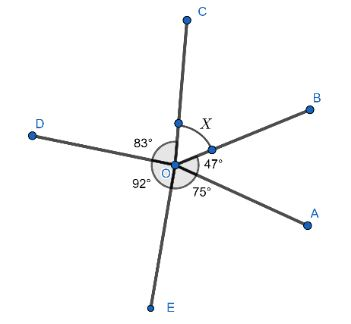
Given, a fig is given in which angles are given.

To find the unknown angle which is given in the angle.
To solve this equation sum of all the angles are equal to
On putting all the values from the given things.
On further adding all the angles.
Taking
On further solving
Hence, the value of the unknown angle is
Note:To solve this type of question you must know the angles made by a single line incomplete revolution and the angle between two string lines and two perpendicular lines. And if all angles are given in terms of a single variable then try to find the value of that single variable. and apply that to all the relations of the angles and find the values of all known angles.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE




