
Answer
457.8k+ views
Hint:
Proceed with the basic definition of given trigonometric ratios and from that we are able to get the idea about the sides of the triangle as if two sides are known then the third side can be calculated using Pythagoras theorem. Hence, knowing the length of all three sides we can obtain various other trigonometric ratios. Also, considering the sign conventions that in the first quadrant all are positive, in the second quadrant sine, cosecant are positive, in third quadrant tangent and cotangent are positive and in the fourth quadrant cosine and secant are positive. And hence considering all these we can obtain our required answer.
Complete step by step solution:
As the given trigonometric ratio is \[\sin x = \dfrac{3}{5}\]
And so in second quadrant we know that only sine and cosecant are positive while all other trigonometric ratios are negative.
Hence, now making triangle in order to get lengths of all sides as,
Diagram:
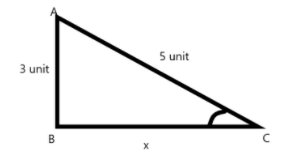
Using Pythagoras theorem calculating the value of x,
Using \[AC = \sqrt {A{B^2} + B{C^2}} \]
On squaring both sides \[A{C^2} = A{B^2} + B{C^2}\]
Now putting the length of side in above equation we can calculate x as,
\[ \Rightarrow \]\[{(5)^2} = {x^2} + {(3)^2}\]
On solving above equation we get,
\[ \Rightarrow \]\[{(x)^2} = 25 - 9\]
On simplifying we get,
\[ \Rightarrow \]\[{(x)^2} = 16\]
Hence, on taking square root,
\[ \Rightarrow \]\[x = 4\,unit\]
Now, as all the side lengths are known we can calculate all trigonometric ratios using proper convention as,
\[ \Rightarrow \]\[\cos x = \dfrac{{adj.}}{{hypo.}} = - \dfrac{4}{5}\]
On calculating other ratio as,
\[ \Rightarrow \]\[\tan x = \dfrac{{opp.}}{{adj.}} = - \dfrac{3}{4}\]
As we know that cosecant, secant and cotangent are inverse of sine, cosine and tangent hence on taking their ratios we get,
\[ \Rightarrow \]\[\cos ecx = \dfrac{{hypo.}}{{opp.}} = \dfrac{5}{3}\]
On calculating another ratio we get,
\[ \Rightarrow \]\[secx = \dfrac{{hypo.}}{{adj.}} = - \dfrac{5}{4}\]
And calculating another ratio we get,
\[ \Rightarrow \]\[\cot x = \dfrac{{adj.}}{{opp.}} = - \dfrac{4}{3}\]
Hence, all the required ratios are calculated.
Note:
There are six trigonometric ratios, sine, cosine, tangent, cosecant, secant and cotangent. These six trigonometric ratios are abbreviated as sin, cos, tan, csc, sec, cot. These are referred to as ratios since they can be expressed in terms of the sides of a right-angled triangle for a specific angle x.
Apply Pythagoras theorem carefully and also draw and calculate the triangle and sides of the triangle without any mistake.
In mathematics, the trigonometric functions are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others.
Proceed with the basic definition of given trigonometric ratios and from that we are able to get the idea about the sides of the triangle as if two sides are known then the third side can be calculated using Pythagoras theorem. Hence, knowing the length of all three sides we can obtain various other trigonometric ratios. Also, considering the sign conventions that in the first quadrant all are positive, in the second quadrant sine, cosecant are positive, in third quadrant tangent and cotangent are positive and in the fourth quadrant cosine and secant are positive. And hence considering all these we can obtain our required answer.
Complete step by step solution:
As the given trigonometric ratio is \[\sin x = \dfrac{3}{5}\]
And so in second quadrant we know that only sine and cosecant are positive while all other trigonometric ratios are negative.
Hence, now making triangle in order to get lengths of all sides as,
Diagram:

Using Pythagoras theorem calculating the value of x,
Using \[AC = \sqrt {A{B^2} + B{C^2}} \]
On squaring both sides \[A{C^2} = A{B^2} + B{C^2}\]
Now putting the length of side in above equation we can calculate x as,
\[ \Rightarrow \]\[{(5)^2} = {x^2} + {(3)^2}\]
On solving above equation we get,
\[ \Rightarrow \]\[{(x)^2} = 25 - 9\]
On simplifying we get,
\[ \Rightarrow \]\[{(x)^2} = 16\]
Hence, on taking square root,
\[ \Rightarrow \]\[x = 4\,unit\]
Now, as all the side lengths are known we can calculate all trigonometric ratios using proper convention as,
\[ \Rightarrow \]\[\cos x = \dfrac{{adj.}}{{hypo.}} = - \dfrac{4}{5}\]
On calculating other ratio as,
\[ \Rightarrow \]\[\tan x = \dfrac{{opp.}}{{adj.}} = - \dfrac{3}{4}\]
As we know that cosecant, secant and cotangent are inverse of sine, cosine and tangent hence on taking their ratios we get,
\[ \Rightarrow \]\[\cos ecx = \dfrac{{hypo.}}{{opp.}} = \dfrac{5}{3}\]
On calculating another ratio we get,
\[ \Rightarrow \]\[secx = \dfrac{{hypo.}}{{adj.}} = - \dfrac{5}{4}\]
And calculating another ratio we get,
\[ \Rightarrow \]\[\cot x = \dfrac{{adj.}}{{opp.}} = - \dfrac{4}{3}\]
Hence, all the required ratios are calculated.
Note:
There are six trigonometric ratios, sine, cosine, tangent, cosecant, secant and cotangent. These six trigonometric ratios are abbreviated as sin, cos, tan, csc, sec, cot. These are referred to as ratios since they can be expressed in terms of the sides of a right-angled triangle for a specific angle x.
Apply Pythagoras theorem carefully and also draw and calculate the triangle and sides of the triangle without any mistake.
In mathematics, the trigonometric functions are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others.
Watch videos on
Find the values of other five trigonometric ratios;
\[\sin x = \dfrac{3}{5}\], x lies in the second quadrant.
\[\sin x = \dfrac{3}{5}\], x lies in the second quadrant.

Trigonometric Functions NCERT EXERCISE 3.2 (Q.2) | Class 11 Maths | Abhishek Sir
Subscribe
 Share
Share likes
44 Views
1 year ago
Recently Updated Pages
10 Examples of Evaporation in Daily Life with Explanations

10 Examples of Diffusion in Everyday Life

1 g of dry green algae absorb 47 times 10 3 moles of class 11 chemistry CBSE

If x be real then the maximum value of 5 + 4x 4x2 will class 10 maths JEE_Main

If the coordinates of the points A B and C be 443 23 class 10 maths JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

In the tincture of iodine which is solute and solv class 11 chemistry CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE



 Watch Video
Watch Video
