
Find the vertical and horizontal component.
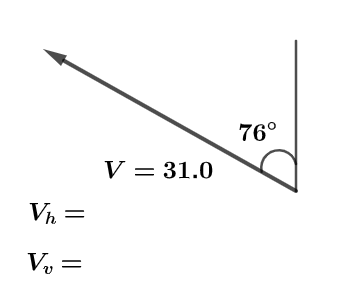

Answer
399.6k+ views
Hint: In order to solve this question, we should know about trigonometric ratios and here we have given a vector which is making an angle of
Formula used: In a right angle triangle, if one of the angle is
Complete step by step solution:
According to the question, we have given the magnitude of a vector V as
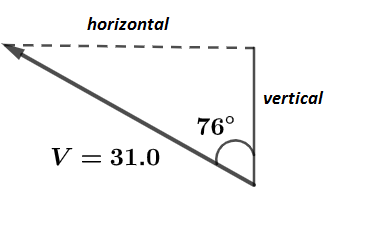
So, for vertical component we will use cosine of the angle made but horizontal component line so,
and similarly for vertical component from the diagram we have,
Hence, the horizontal and vertical components of given vectors are
Note:
It should be remembered that, while using trigonometric ratios, always check the adjacent side is one with which hypotenuse makes an angle and here, the vector’s magnitude has not any units so, we simply write in terms of numerical values, a vector can represent either velocity, acceleration or any other vector physical quantity.
Formula used: In a right angle triangle, if one of the angle is
Complete step by step solution:
According to the question, we have given the magnitude of a vector V as

So, for vertical component we will use cosine of the angle made but horizontal component line so,
and similarly for vertical component from the diagram we have,
Hence, the horizontal and vertical components of given vectors are
Note:
It should be remembered that, while using trigonometric ratios, always check the adjacent side is one with which hypotenuse makes an angle and here, the vector’s magnitude has not any units so, we simply write in terms of numerical values, a vector can represent either velocity, acceleration or any other vector physical quantity.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

Find the missing frequency in the following distribution class 11 maths CBSE

Difference between physical and chemical change class 11 chemistry CBSE

Whales are warmblooded animals which live in cold seas class 11 biology CBSE

Define least count of vernier callipers How do you class 11 physics CBSE




