
How do you find the vertical asymptote of a logarithmic function?
Answer
463.8k+ views
Hint: Horizontal asymptote is the value that any function in x-variable approaches infinity x approaches infinity and as x approaches negative infinity. Vertical asymptotes occur wherever the value of function approaches infinity or negative infinity. Keeping this information in mind, we shall proceed to find the vertical asymptote of a logarithmic function.
Complete step-by-step solution:
Vertical asymptote is basically an invisible vertical line that the graph of a function approaches and gets really close to but never actually touches. The point that the graph of a function never touches is the point where it is undefined.
We know that the logarithmic function is not defined at
The graph of
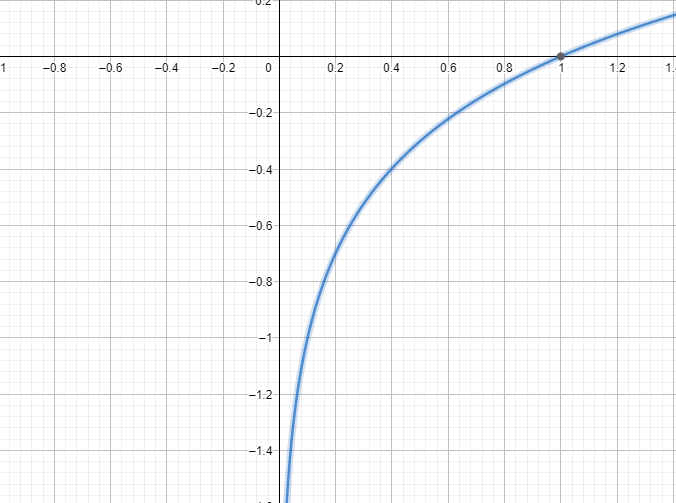
Even though the function seems to be approaching the y-axis but it never touches it in real. Therefore, the vertical asymptote of a logarithmic function is the y-axis.
Note: As we approach from the left, a vertical asymptote looks like the graph is approaching a vertical up to infinity but it never intersects with any point on the x-axis as it is not defined at that point. Similarly, as we approach from the right, the graph again does not intersect at any point and approaches a vertical up to infinity as it is undefined at that same point.
Complete step-by-step solution:
Vertical asymptote is basically an invisible vertical line that the graph of a function approaches and gets really close to but never actually touches. The point that the graph of a function never touches is the point where it is undefined.
We know that the logarithmic function is not defined at
The graph of

Even though the function seems to be approaching the y-axis but it never touches it in real. Therefore, the vertical asymptote of a logarithmic function is the y-axis.
Note: As we approach from the left, a vertical asymptote looks like the graph is approaching a vertical up to infinity but it never intersects with any point on the x-axis as it is not defined at that point. Similarly, as we approach from the right, the graph again does not intersect at any point and approaches a vertical up to infinity as it is undefined at that same point.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




